mirror of
https://gitee.com/TheAlgorithms/LeetCodeAnimation.git
synced 2024-12-06 15:19:44 +08:00
commit
a1a7ffaba3
Binary file not shown.
Binary file not shown.
BIN
0024-Swap-Nodes-in-Pairs/Animation/Animation1.gif
Normal file
BIN
0024-Swap-Nodes-in-Pairs/Animation/Animation1.gif
Normal file
Binary file not shown.
|
After Width: | Height: | Size: 4.7 MiB |
BIN
0024-Swap-Nodes-in-Pairs/Animation/Animation2.gif
Normal file
BIN
0024-Swap-Nodes-in-Pairs/Animation/Animation2.gif
Normal file
Binary file not shown.
|
After Width: | Height: | Size: 2.4 MiB |
128
0024-Swap-Nodes-in-Pairs/Article/0024-Swap-Nodes-in-Pairs2.md
Normal file
128
0024-Swap-Nodes-in-Pairs/Article/0024-Swap-Nodes-in-Pairs2.md
Normal file
@ -0,0 +1,128 @@
|
||||
# LeetCode 第 24 号问题:两两交换链表中的节点
|
||||
|
||||
> 本文首发于公众号「图解面试算法」,是 [图解 LeetCode ](<https://github.com/MisterBooo/LeetCodeAnimation>) 系列文章之一。
|
||||
>
|
||||
> 同步博客:https://www.algomooc.com
|
||||
|
||||
题目来源于 LeetCode 上第 24 号问题:两两交换链表中的节点。
|
||||
|
||||
### 题目描述
|
||||
|
||||
给定一个链表,两两交换其中相邻的节点,并返回交换后的链表。
|
||||
|
||||
**你不能只是单纯的改变节点内部的值**,而是需要实际的进行节点交换。
|
||||
|
||||
**示例:**
|
||||
|
||||
```
|
||||
给定 1->2->3->4, 你应该返回 2->1->4->3.
|
||||
```
|
||||
|
||||
### 题目解析 - 迭代法
|
||||
|
||||
由题目描述可知需要两两交换, 那么以两个为一组子链表交换指针即可, 在设置一个 **哨兵** 指向交换后的子链表 (或者哨兵提前指向子链表的第二个节点,因为第二个节点交换后就成了第一个节点); 然后让哨兵指向下一组子链表,继续交换,直至最后.
|
||||
|
||||
设 **哨兵** 为 节点 `prev`, 子链表第一个节点为 `A`, 第二个节点为 `B`, 第三个节点为 `C`, 那么操作流程如下:
|
||||
|
||||
- 终止条件 `head == null && head -> next == null`
|
||||
1. prev -> B ( A -> B -> C )
|
||||
2. A - > C
|
||||
3. B -> A ( prev -> B -> A -> C )
|
||||
4. prev -> A
|
||||
5. head -> C
|
||||
6. 循环以上步骤
|
||||
|
||||
### 动画描述
|
||||
|
||||
<img src="../Animation/Animation1.gif" alt="Animation1" style="zoom:150%;" />
|
||||
|
||||
### 代码实现
|
||||
|
||||
```javascript
|
||||
/**
|
||||
* JavaScript描述
|
||||
* 迭代法
|
||||
*/
|
||||
var swapPairs = function(head) {
|
||||
let dummy = new ListNode(0);
|
||||
dummy.next = head;
|
||||
|
||||
let prevNode = dummy;
|
||||
|
||||
while (head !== null && head.next !== null) {
|
||||
// Nodes to be swapped
|
||||
let firstNode = head,
|
||||
secondNode = head.next;
|
||||
// Swapping
|
||||
prevNode.next = secondNode; // 放到交换前后都可以
|
||||
firstNode.next = secondNode.next;
|
||||
secondNode.next = firstNode;
|
||||
// Reinitializing the head and prevNode for next swap
|
||||
prevNode = firstNode;
|
||||
head = firstNode.next;
|
||||
}
|
||||
return dummy.next;
|
||||
};
|
||||
```
|
||||
|
||||
### 复杂度分析
|
||||
|
||||
- 时间复杂度:**O(N)**,其中 *N* 指的是链表的节点数量
|
||||
- 空间复杂度:**O(1)**
|
||||
|
||||
### 题目解析 - 递归
|
||||
|
||||
递归的思路和迭代类似, 都是分组交换. 具体来说这里的递归不是针对一个问题深入进去,而是不断向后推进.
|
||||
|
||||
- 每次递归只交换一对节点
|
||||
- 下一次递归则是交换下一对节点
|
||||
- 交换完成后返回第二个节点, 因为它是交换后的子链表新头
|
||||
- 递归完成后返回第一次递归的第二个节点, 这就是新链表的头结点
|
||||
|
||||
**注意:** 不要人肉递归, 更多关注整体逻辑
|
||||
|
||||
示例执行大致流程为:
|
||||
|
||||
- 终止条件: `(head == null) || (head.next == null)`
|
||||
1. 1 -> 2 -> 3 -> 4 ( 原始链表 )
|
||||
2. 1 -> 3 -> 4
|
||||
3. ( 2 -> 1 ) -> 3 -> 4 ( 第一次递归完成后返回原来的第二个节点, 也就是值为 2 的节点 )
|
||||
4. 2 -> 1 -> 3 -> null
|
||||
5. 2 -> 1 -> ( 4 -> 3 ) ( 第二次递归完成后返回原来的第二个节点, 也就是值为 4 的节点 )
|
||||
|
||||
### 动画描述
|
||||
|
||||
<img src="../Animation/Animation2.gif" alt="Animation2" style="zoom:150%;" />
|
||||
|
||||
### 代码实现
|
||||
|
||||
```javascript
|
||||
/**
|
||||
* JavaScript描述
|
||||
* 递归法
|
||||
*/
|
||||
var swapPairs = function(head) {
|
||||
if (head == null || head.next == null) {
|
||||
return head;
|
||||
}
|
||||
// Nodes to be swapped
|
||||
let firstNode = head,
|
||||
secondNode = head.next;
|
||||
// Swapping
|
||||
firstNode.next = swapPairs(secondNode.next);
|
||||
secondNode.next = firstNode;
|
||||
|
||||
return secondNode;
|
||||
|
||||
};
|
||||
```
|
||||
|
||||
### 复杂度分析
|
||||
|
||||
- 时间复杂度:**O(N)**,其中 *N* 指的是链表的节点数量
|
||||
- 空间复杂度:**O(N)**, 递归过程使用的堆栈空间
|
||||
|
||||
|
||||
|
||||

|
||||
|
||||
Binary file not shown.
BIN
0142-Linked-List-Cycle-ii/Animation/Animation.gif
Normal file
BIN
0142-Linked-List-Cycle-ii/Animation/Animation.gif
Normal file
Binary file not shown.
|
After Width: | Height: | Size: 5.1 MiB |
176
0142-Linked-List-Cycle-ii/Article/0142-Linked-List-Cycle-ii.md
Normal file
176
0142-Linked-List-Cycle-ii/Article/0142-Linked-List-Cycle-ii.md
Normal file
@ -0,0 +1,176 @@
|
||||
# LeetCode 第 142 号问题:环形链表 II
|
||||
|
||||
> 本文首发于公众号「图解面试算法」,是 [图解 LeetCode ](<https://github.com/MisterBooo/LeetCodeAnimation>) 系列文章之一。
|
||||
>
|
||||
> 同步博客:https://www.algomooc.com
|
||||
|
||||
今天分享的题目来源于 LeetCode 上第 142 号问题:环形链表II。题目难度为 Medium 。
|
||||
|
||||
### 题目描述
|
||||
|
||||
给定一个链表,返回链表开始入环的第一个节点。 如果链表无环,则返回 `null`。
|
||||
|
||||
为了表示给定链表中的环,我们使用整数 `pos` 来表示链表尾连接到链表中的位置(索引从 0 开始)。 如果 `pos` 是 `-1`,则在该链表中没有环。
|
||||
|
||||
**示例 1:**
|
||||
|
||||
```
|
||||
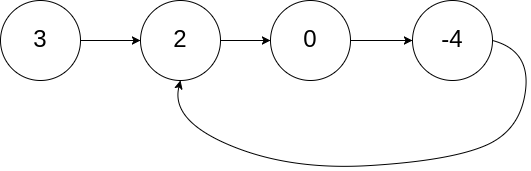
输入:head = [3,2,0,-4], pos = 1
|
||||
输出:tail connects to node index 1
|
||||
解释:链表中有一个环,其尾部连接到第二个节点。
|
||||
```
|
||||
|
||||
|
||||
|
||||
**示例 2:**
|
||||
|
||||
```
|
||||
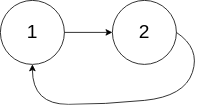
输入:head = [1,2], pos = 0
|
||||
输出:tail connects to node index 0
|
||||
解释:链表中有一个环,其尾部连接到第一个节点。
|
||||
```
|
||||
|
||||
|
||||
|
||||
**示例 3:**
|
||||
|
||||
```
|
||||
输入:head = [1], pos = -1
|
||||
输出:no cycle
|
||||
解释:链表中没有环。
|
||||
```
|
||||
|
||||

|
||||
|
||||
**进阶:**
|
||||
|
||||
你是否可以不用额外空间解决此题?
|
||||
|
||||
### 题目解析 - 哈希表
|
||||
|
||||
普通解法就是利用哈希表保存访问过的节点, 同时遍历过程中检查哈希表中是否已存在相同的节点
|
||||
|
||||
### 代码实现
|
||||
|
||||
```javascript
|
||||
/**
|
||||
* JavaScript 描述
|
||||
* 哈希表方法
|
||||
*/
|
||||
var detectCycle = function(head) {
|
||||
let res = [ ];
|
||||
while (head !== null) {
|
||||
if (res.includes(head)) {
|
||||
return head;
|
||||
}
|
||||
res.push(head);
|
||||
head = head.next;
|
||||
}
|
||||
return null;
|
||||
};
|
||||
```
|
||||
|
||||
### 复杂度分析
|
||||
|
||||
- 时间复杂度:**O(n)**
|
||||
- 空间复杂度:**O(n)**
|
||||
|
||||
### 题目解析 - Floyd 算法
|
||||
|
||||
Floyd算法 可以达到常量空间解决此问题.
|
||||
|
||||
我在维基百科找到了这个算法描述, 在此引用一下.
|
||||
|
||||
**Floyd判圈算法**(**Floyd Cycle Detection Algorithm**),又称 **龟兔赛跑算法**(**Tortoise and Hare Algorithm**),是一个可以在[有限状态机](https://zh.wikipedia.org/wiki/有限状态机)、[迭代函数](https://zh.wikipedia.org/wiki/迭代函数)或者[链表](https://zh.wikipedia.org/wiki/链表)上判断是否存在[环](https://zh.wikipedia.org/wiki/環_(圖論)),求出该环的起点与长度的算法。
|
||||
|
||||
如果有限状态机、迭代函数或者链表存在环,那么一定存在一个起点可以到达某个环的某处 ( 这个起点也可以在某个环上 )。
|
||||
|
||||
初始状态下,假设已知某个起点节点为节点 *S*。现设两个指针 `t` 和 `h` ,将它们均指向 *S*。
|
||||
|
||||
接着,同时让 `t` 和 `h` 往前推进,但是二者的速度不同:`t` 每前进 `1` 步, `h` 前进 `2` 步。只要二者都可以前进而且没有相遇,就如此保持二者的推进。当 `h` 无法前进,即到达某个没有后继的节点时,就可以确定从 *S* 出发不会遇到环。反之当 `t` 与 `h` 再次相遇时,就可以确定从 S 出发一定会进入某个环,设其为环 *C*。
|
||||
|
||||
如果确定了存在某个环,就可以求此环的起点与长度。
|
||||
|
||||
上述算法刚判断出存在环 *C* 时,显然 t 和 `h` 位于同一节点,设其为节点 *M*。显然,仅需令 `h` 不动,而t不断推进,最终又会返回节点 *M*,统计这一次t推进的步数,显然这就是环 *C* 的长度。
|
||||
|
||||
为了求出环 *C* 的起点,只要令h仍均位于节点 *M* ,而令t返回起点节点 *S* ,此时h与t之间距为环 *C* 长度的整数倍。随后,同时让 `t` 和 `h` 往前推进,且保持二者的速度相同:`t` 每前进 `1` 步,`h` 前进 `1` 步。持续该过程直至 `t` 与 `h` 再一次相遇,设此次相遇时位于同一节点 *P*,则节点 *P* 即为从节点 *S* 出发所到达的环 *C* 的第一个节点,即环 *C* 的一个起点。
|
||||
|
||||
**看完之后是不是很多疑点, 觉得为什么会这样呢?**
|
||||
|
||||
下面用数学简单证明一下
|
||||
|
||||
假设 链表的节点数为 `num`, 从 head 到链表环入口节点数为 `m` (不包含入口节点), 环的节点数为 `n`, 链表环入口设点为 *P*
|
||||
|
||||
由此可得 `num = m + n`
|
||||
|
||||
假设 慢指针 `Tortoise` (乌龟) 每次走 `1` 个节点, 走了 `x` 步
|
||||
|
||||
假设 快指针 `Hare` (兔子) 每次走 `2` 个节点, 走了 `f` 步
|
||||
|
||||
那么 `f = 2x`
|
||||
|
||||
当第一次相遇时, 必然是在环内, 设其点为 *M*, 兔子第一次到达 *M* 点后至少又在环内饶了一圈后追上乌龟,
|
||||
|
||||
假设绕了 `k` 圈, 那么可以得到
|
||||
|
||||
`f = x + kn`
|
||||
|
||||
兔子到达 *P* 点的步数为
|
||||
|
||||
`f = m + kn`
|
||||
|
||||
由 `f = 2x` 和 `f = x + kn` 两个等式可以得到 `x = kn`
|
||||
|
||||
由 `f = m + kn` 和 `x = kn` 可知, 乌龟到达 *P* 点还需要走 `m` 步
|
||||
|
||||
而 `m` 的长度正是从 head 到链表环入口节点数的长度, 这是未知的,
|
||||
|
||||
那么让兔子从 head 以乌龟的速度走, 乌龟在 *M* 点走, 当兔子和乌龟相遇时即走了 `m` 步, 也就到达了 *P* 节点.
|
||||
|
||||
### 动画描述
|
||||
|
||||

|
||||
|
||||
### 代码实现
|
||||
|
||||
```java
|
||||
/**
|
||||
* JavaScript 描述
|
||||
* Floyd判圈算法
|
||||
*/
|
||||
var detectCycle = function(head) {
|
||||
if (head == null) {
|
||||
return head;
|
||||
}
|
||||
// 设置快慢指针
|
||||
let tortoise = head,
|
||||
hare = head;
|
||||
// 检查链表是否有环
|
||||
while (true) {
|
||||
if (hare == null || hare.next == null) {
|
||||
return null;
|
||||
}
|
||||
hare = hare.next.next;
|
||||
tortoise = tortoise.next;
|
||||
if (hare == tortoise) {
|
||||
break;
|
||||
}
|
||||
}
|
||||
// 兔子和乌龟第二次相遇找到环入口
|
||||
hare = head;
|
||||
while (hare != tortoise) {
|
||||
hare = hare.next;
|
||||
tortoise = tortoise.next;
|
||||
}
|
||||
return hare;
|
||||
};
|
||||
```
|
||||
|
||||
### 复杂度分析
|
||||
|
||||
- 时间复杂度:**O(n)**
|
||||
- 有环情况下, 第一次和第二次相遇, 乌龟步数都小于链表节点数, 因此与链表节点数成线性关系;
|
||||
- 无环情况下, 兔子大约需要 n/2 步数到达最后, 因此也与链表节点数成线性关系.
|
||||
- 空间复杂度:**O(1)** , 双指针使用常数大小的额外空间
|
||||
|
||||

|
||||
Loading…
Reference in New Issue
Block a user