Algorithms/algo
1
0
Fork 0
Code Issues Pull Requests Actions Packages Projects Releases Wiki Activity

Merge remote-tracking branch 'c-cpp/master'

Browse Source 
111
This commit is contained in:
jinshaohui 2018-10-31 20:22:13 +08:00
commit 17c5b96478
55 changed files with 3299 additions and 126 deletions
Show Stats Download Patch File Download Diff File Expand all files Collapse all files

133
c-cpp/07_linkedlist/SingleList.cpp
View File

@ -6,7 +6,7 @@ using namespace std;
class CElement;
/***
* @brief 单链表容器
* @brief 单链表容器
*/
class CSingleList
{
@ -15,61 +15,61 @@ public:
~CSingleList();
/**
* @brief 插入..链表末尾插入
* @return 成功返回非空指针,否则失败
* @brief 插入..链表末尾插入
* @return 成功返回非空指针,否则失败
*/
CElement* Insert(void* lpData, int iDataSize);
/**
* @brief 插入..链表指定位置插入
* @return 成功返回非空指针,否则失败
* @brief 插入..链表指定位置插入
* @return 成功返回非空指针,否则失败
*/
CElement* Insert(CElement* lpElement, void* lpData, int iDataSize);
/**
* @brief 删除
* @brief 删除
*/
void Delete(CElement*);
/**
* @brief 链首
* @brief 链首
*/
CElement* Begin();
/**
* @brief 下一个元素
* @brief 下一个元素
*/
CElement* Next();
/***
* @brief 链尾
* @brief 链尾
*/
CElement* End();
/**
* @brief 是否是空链表
* @return 空返回TRUE，否则返回FALSE
* @brief 是否是空链表
* @return 空返回TRUE，否则返回FALSE
*/
bool Empty();
/**
* @brief 反转
* @brief 反转
*/
void Reverse();
/**
* @brief 检测环
* @return 返回TRUE时表示链表存在环,否则不存在环.
* @brief 检测环
* @return 返回TRUE时表示链表存在环,否则不存在环.
*/
bool CheckCircle();
/**
* @brief 合并2个有序的链表
* @brief 合并2个有序的链表
*/
void Merge(CSingleList& lst, std::function<int(void* t1, void* t2)>);
/**
* @brief 删除倒数第K个结点
* @brief 删除倒数第K个结点
*/
void DeleteLastKth(int k);
/**
* @brief 求中间节点
* @brief 求中间节点
*/
CElement* Center();
private:
@ -80,18 +80,18 @@ private:
CSingleList(CSingleList const & rhs);
CSingleList& operator= (CSingleList const& rhs);
private:
/**头结点*/
/**头结点*/
CElement* m_lpHead;
/**哨兵*/
/**哨兵*/
CElement* m_lpSentinel;
/**空结点，用于End()返回 */
/**空结点，用于End()返回 */
CElement* m_lpNull;
/**当前结点. 枚举时使用. */
/**当前结点. 枚举时使用. */
CElement* m_lpCur;
};
/***
* @brief 单链表结点元素.
* @brief 单链表结点元素.
*/
class CElement
{
@ -101,11 +101,11 @@ protected:
~CElement();
public:
/***
* @brief 获取数据指针
* @brief 获取数据指针
*/
void* GetDataPtr();
protected:
/**下一个结点*/
/**下一个结点*/
CElement* m_lpNext;
void* m_lpData;
};
@ -113,7 +113,7 @@ protected:
void CreateList(CSingleList& lst)
{
//循环插入元素到链表尾
//循环插入元素到链表尾
for(int i=1; i<10;i++)
{
int* p = new int();
@ -134,15 +134,15 @@ void PrintList(CSingleList& lst)
int main()
{
{
/// 链表的基本操作，插入/枚举/删除
/// 链表的基本操作，插入/枚举/删除
CSingleList lst;
CElement* lpElement = NULL;
CreateList(lst);
std::cout<<"枚举链表当前的元素"<<std::endl;
std::cout<<"枚举链表当前的元素"<<std::endl;
PrintList(lst);
std::cout<<"查找指定元素,并在指定元素后面插入新元素"<<std::endl;
std::cout<<"查找指定元素,并在指定元素后面插入新元素"<<std::endl;
lpElement = lst.Begin();
while(lpElement != lst.End())
{
@ -157,10 +157,10 @@ int main()
}
}
std::cout<<"枚举链表当前的元素"<<std::endl;
std::cout<<"枚举链表当前的元素"<<std::endl;
PrintList(lst);
std::cout<<"查找指定元素(数字是7的元素),并删除指定元素"<<std::endl;
std::cout<<"查找指定元素(数字是7的元素),并删除指定元素"<<std::endl;
lpElement = lst.Begin();
while(lpElement != lst.End())
{
@ -172,52 +172,52 @@ int main()
lpElement = lst.Next();
}
}
std::cout<<"枚举链表当前的元素"<<std::endl;
std::cout<<"枚举链表当前的元素"<<std::endl;
PrintList(lst);
}
std::cout<<"--------------------------"<<std::endl;
{
/// 链表的反转
/// 链表的反转
CSingleList lst;
CElement* lpElement = NULL;
CreateList(lst);
std::cout<<"反转"<<std::endl;
std::cout<<"反转"<<std::endl;
lst.Reverse();
PrintList(lst);
}
std::cout<<"--------------------------"<<std::endl;
{
/// 检测环
/// 检测环
CSingleList lst;
CElement* lpElement = NULL;
CreateList(lst);
std::cout<<"检测环"<<std::endl;
std::cout<<"检测环"<<std::endl;
bool bRet = lst.CheckCircle();
if(bRet){
std::cout<<"存在环."<<std::endl;
std::cout<<"存在环."<<std::endl;
}else{
std::cout<<"不存在环."<<std::endl;
std::cout<<"不存在环."<<std::endl;
}
}
std::cout<<"--------------------------"<<std::endl;
{
/// 有序链表合并
/// 有序链表合并
CSingleList lst,lst2;
CElement* lpElement = NULL;
for(int i=1; i<10;i++)
for(int i=1; i<30;i++)
{
int* p = new int();
*p = i;
if(i%2){
if(i%4){
lst2.Insert(p, 4);
}else{
lst.Insert(p, 4);
}
}
std::cout<<"枚举链表当前的元素"<<std::endl;
std::cout<<"枚举链表当前的元素"<<std::endl;
PrintList(lst);
std::cout<<"......"<<std::endl;
PrintList(lst2);
@ -231,31 +231,31 @@ int main()
}
return 0;
});
std::cout<<"合并之后，打印当前链表."<<std::endl;
std::cout<<"合并之后，打印当前链表."<<std::endl;
PrintList(lst);
}
std::cout<<"--------------------------"<<std::endl;
{
/// 删除倒数第K个结点,并查看中间节点
/// 删除倒数第K个结点,并查看中间节点
CSingleList lst;
CreateList(lst);
std::cout<<"删除倒数第0个结点"<<std::endl;
std::cout<<"删除倒数第0个结点"<<std::endl;
lst.DeleteLastKth(0);
PrintList(lst);
CElement* lpCenter = lst.Center();
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
std::cout<<"删除倒数第1个结点"<<std::endl;
std::cout<<"删除倒数第1个结点"<<std::endl;
lst.DeleteLastKth(1);
PrintList(lst);
lpCenter = lst.Center();
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
std::cout<<"删除倒数第3个结点"<<std::endl;
std::cout<<"删除倒数第3个结点"<<std::endl;
lst.DeleteLastKth(3);
PrintList(lst);
lpCenter = lst.Center();
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
std::cout<<"中间节点:"<<*((int*)lpCenter->GetDataPtr())<<std::endl;
}
std::cin.ignore();
@ -316,10 +316,10 @@ CElement* CSingleList::Insert(CElement* lpElement, void* lpData, int iDataSize)
}
void CSingleList::Insert(CElement* lpNewElement, CElement* lpCurElement, bool bBack /*= true*/)
{
if(bBack){//插入到指定元素的后面
if(bBack){//插入到指定元素的后面
lpNewElement->m_lpNext = lpCurElement->m_lpNext;
lpCurElement->m_lpNext = lpNewElement;
}else{//插入到指定元素的前面
}else{//插入到指定元素的前面
CElement* lpIter = m_lpSentinel;
while(NULL != lpIter)
{
@ -443,11 +443,13 @@ bool CSingleList::CheckCircle()
return false;
}
/**
* 合并的2个链表必须是有序的
*/
void CSingleList::Merge(CSingleList& lst, std::function<int(void* t1, void* t2)> fnCompare)
{
CElement* lpL1 = Begin();
CElement* lpL2 = lst.Begin();
CElement* lpTail = NULL;
if(!fnCompare)
{
@ -458,7 +460,26 @@ void CSingleList::Merge(CSingleList& lst, std::function<int(void* t1, void* t2)>
{
if(lpL1 != End())
{
/**
* 查找需要插入的正确位置
*
* 链表1,链表2; 链表1 <- 链表2, 链表2被合并到链表1中
*
* 如果链表1的元素小于链表2中的元素，则循环查找链表1中大于链表2中的当前元素的元素
* 如果在链表1中找到满足上面条件的的元素位置[A]时，则把链表2中的当前元素插入到元素位置[A]的前面;
* 如果在链表1中不存在这个位置则在链表1的末位插入元素
*/
iRet = fnCompare(lpL1->GetDataPtr(), lpL2->GetDataPtr());
if(iRet < 0){
lpL1 = Next();
while(lpL1 != End()){
iRet = fnCompare(lpL1->GetDataPtr(), lpL2->GetDataPtr());
if(iRet > 0){
break;
}
lpL1 = Next();
}
}
}else{
iRet = -1;
}
@ -468,17 +489,13 @@ void CSingleList::Merge(CSingleList& lst, std::function<int(void* t1, void* t2)>
lpNewElement->m_lpData = lpL2->GetDataPtr();
if(lpL1 != End())
{
Insert(lpNewElement,lpL1, iRet <= 0);
Insert(lpNewElement,lpL1, iRet < 0);
}else{
if(NULL == lpTail)
{
lpTail = Tail();
}
CElement* lpTail = Tail();
Insert(lpNewElement,lpTail);
}
}
lpL2 = lst.Next();
lpL1 = Next();
}
}

0
c-cpp/13_sorts/.gitkeep Normal file
View File

43
c-cpp/13_sorts/bucket_sort.hpp Normal file
View File

@ -0,0 +1,43 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#ifndef SORTS_BUCKET_SORT_HPP_
#define SORTS_BUCKET_SORT_HPP_
#include <iterator>
#include <functional>
#include <algorithm>
#include <vector>
template <size_t BucketSize,
typename IterT,
typename T = typename std::iterator_traits<IterT>::value_type,
typename Compare = std::less<T>>
void bucket_sort(IterT first, IterT last, Compare comp = Compare()) {
const T min = *std::min_element(first, last), max = *std::max_element(first, last);
const T range = max + 1 - min;
const size_t bucket_num = (range - 1) / BucketSize + 1;
std::vector<std::vector<T>> buckets(bucket_num);
for (auto b : buckets) {
b.reserve(2 * BucketSize);
}
for (IterT i = first; i != last; ++i) {
size_t idx = (*i - min) / BucketSize;
buckets[idx].emplace_back(*i);
}
IterT dest = first;
for (auto b : buckets) {
std::sort(b.begin(), b.end(), comp);
std::copy(b.begin(), b.end(), dest);
dest += b.size();
}
return;
}
#endif // SORTS_BUCKET_SORT_HPP_

33
c-cpp/13_sorts/bucket_sort_test.cc Normal file
View File

@ -0,0 +1,33 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#include <iostream>
#include <vector>
#include <functional>
#include "bucket_sort.hpp"
template <size_t BucketSize,
typename Container,
typename T = typename Container::value_type,
typename Compare = std::less<T>>
void test_bucket_sort(Container cont, Compare comp = Compare()) {
bucket_sort<BucketSize>(cont.begin(), cont.end(), comp);
std::transform(cont.begin(), cont.end(), std::ostream_iterator<T>(std::cout, " "),
[](T i){ return i; });
std::cout << std::endl;
}
int main() {
std::vector<int> test{3, 1, 4, 1, 5, 9, 2, 6, 5, 3, 5, 8, 9, 7, 9};
test_bucket_sort<2>(test); // 1 1 2 3 3 4 5 5 5 6 7 8 9 9 9
test_bucket_sort<3>(test); // 1 1 2 3 3 4 5 5 5 6 7 8 9 9 9
test_bucket_sort<4>(test); // 1 1 2 3 3 4 5 5 5 6 7 8 9 9 9
test_bucket_sort<5>(test); // 1 1 2 3 3 4 5 5 5 6 7 8 9 9 9
test_bucket_sort<6>(test); // 1 1 2 3 3 4 5 5 5 6 7 8 9 9 9
return 0;
}

40
c-cpp/13_sorts/counting_sort.hpp Normal file
View File

@ -0,0 +1,40 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#ifndef SORTS_COUNTING_SORT_HPP_
#define SORTS_COUNTING_SORT_HPP_
#include <iterator>
#include <functional>
#include <algorithm>
#include <vector>
template <typename IterT,
typename T = typename std::iterator_traits<IterT>::value_type>
void counting_sort(IterT first, IterT last) {
const auto len = std::distance(first, last);
if (len < 2) { return; }
const T max = *std::max_element(first, last);
if (max == 0) { return; }
std::vector<size_t> counter(max + 1);
for (IterT i = first; i != last; ++i) {
++counter[*i];
}
for (size_t i = 1; i != max + 1; ++i) {
const size_t j = max - i;
counter[j] += counter[j + 1]; // Liam Huang: count of numbers that is not less than j.
}
std::vector<T> temp(len);
for (IterT i = first; i != last; ++i) {
temp[len - counter[*i]] = *i;
--counter[*i]; // Liam Huang: stable for relative position.
}
std::copy(temp.begin(), temp.end(), first);
}
#endif // SORTS_COUNTING_SORT_HPP_

36
c-cpp/13_sorts/counting_sort_test.cc Normal file
View File

@ -0,0 +1,36 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#include <iostream>
#include <vector>
#include <functional>
#include "counting_sort.hpp"
template <typename Container,
typename T = typename Container::value_type>
void test_counting_sort(Container cont) {
counting_sort(cont.begin(), cont.end());
std::transform(cont.begin(), cont.end(), std::ostream_iterator<T>(std::cout, " "),
[](T i){ return i; });
std::cout << std::endl;
}
int main() {
// Liam Huang: pi for test
const std::vector<int> test1{3, 1, 4, 1, 5, 9, 2, 6, 5, 3, 5, 8, 9, 7, 9, 3};
const std::vector<int> test2{2, 3, 8, 4, 6, 2, 6, 4, 3, 3, 8, 3, 2, 7, 9};
const std::vector<int> test3{5, 0, 2, 8, 8, 4, 1, 9, 7, 1, 6, 9, 3, 9, 9};
const std::vector<int> test4{3, 7, 5, 1, 0, 5, 8, 2, 0, 9, 7, 4, 9, 4, 4};
const std::vector<int> test5{5, 9, 2, 3, 0, 7, 8, 1, 6, 4, 0, 6, 2, 8, 6};
test_counting_sort(test1); // 1 1 2 3 3 3 4 5 5 5 6 7 8 9 9 9
test_counting_sort(test2); // 2 2 2 3 3 3 3 4 4 6 6 7 8 8 9
test_counting_sort(test3); // 0 1 1 2 3 4 5 6 7 8 8 9 9 9 9
test_counting_sort(test4); // 0 0 1 2 3 4 4 4 5 5 7 7 8 9 9
test_counting_sort(test5); // 0 0 1 2 2 3 4 5 6 6 6 7 8 8 9
return 0;
}

96
c-cpp/15_bsearch/binary_search.c Normal file
View File

@ -0,0 +1,96 @@
#include <stdio.h>
#include <stdlib.h>
#include <stdbool.h>
#include <string.h>
typedef int(*bs)(int *arr, int size, int val);
int binary_search_r(int *arr, int size, int val)
{
int mid = size / 2;
int idx;
if (arr[mid] == val)
return mid;
// mid == 0 means size == 1
// so the only element in array doesn't equal to val
if (!mid)
return -1;
if (arr[mid] < val) {
idx = binary_search_r(arr + mid + 1, size - mid - 1, val);
if (idx != -1)
idx += mid + 1;
} else {
idx = binary_search_r(arr, mid, val);
}
return idx;
}
int binary_search_i(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
if (arr[mid] == val)
return mid;
if (arr[mid] < val)
low = mid + 1;
else
high = mid - 1;
}
return -1;
}
void iteratioin_test(bs binary_search)
{
int arr[10] = {1, 4, 5, 9, 12, 19, 21, 28, 31, 36};
int idx;
idx = binary_search(arr, 10, 12);
if (idx != -1)
printf("find 12 at %d\n", idx);
else
printf("12 not in arr \n");
idx = binary_search(arr, 10, 13);
if (idx != -1)
printf("find 13 at %d\n", idx);
else
printf("13 not in arr \n");
idx = binary_search(arr, 10, 1);
if (idx != -1)
printf("find 1 at %d\n", idx);
else
printf("1 not in arr \n");
idx = binary_search(arr, 10, 36);
if (idx != -1)
printf("find 36 at %d\n", idx);
else
printf("36 not in arr \n");
idx = binary_search(arr, 10, 31);
if (idx != -1)
printf("find 31 at %d\n", idx);
else
printf("31 not in arr \n");
}
int main()
{
printf("=== Test iteration version:\n");
iteratioin_test(binary_search_i);
printf("=== Test recursive version:\n");
iteratioin_test(binary_search_r);
return 0;
}

37
c-cpp/15_bsearch/bsearch.hpp
View File

@ -8,19 +8,16 @@
#include <iterator>
#include <functional>
enum class BsearchPolicy { FIRST, LAST, UNSPECIFIED };
// Liam Huang: The algorithm works right with iterators that meet the ForwardIterator requirement,
// but with a bad time complexity. For better performance, iterators should meet
// the RandomAccessIterator requirement.
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare>
typename Compare = std::less<ValueT>>
IterT bsearch(IterT first,
IterT last,
ValueT target,
Compare comp,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
Compare comp = Compare()) {
IterT result = last;
while (std::distance(first, last) > 0) {
IterT mid = first + std::distance(first, last) / 2;
@ -29,38 +26,12 @@ IterT bsearch(IterT first,
} else if (comp(target, *mid)) {
last = mid;
} else { // equal
if (policy == BsearchPolicy::FIRST) {
if (mid == first or comp(*(mid - 1), *mid)) {
result = mid;
break;
} else {
last = mid;
}
} else if (policy == BsearchPolicy::LAST) {
if (std::distance(mid, last) == 1 or comp(*mid, *(mid + 1))) {
result = mid;
break;
} else {
first = mid + 1;
}
} else {
result = mid;
break;
}
result = mid;
break;
}
}
return result;
}
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare = std::less<ValueT>>
IterT bsearch(IterT first,
IterT last,
ValueT target,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
return bsearch(first, last, target, Compare(), policy);
}
#endif // BSEARCH_BSEARCH_HPP_

14
c-cpp/15_bsearch/bsearch_test.cc
View File

@ -8,10 +8,8 @@
#include "bsearch.hpp"
template <typename VecT, typename T = typename VecT::value_type>
void test_bsearch(const VecT& test,
T target,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
auto it = bsearch(test.begin(), test.end(), target, policy);
void test_bsearch(const VecT& test, T target) {
auto it = bsearch(test.begin(), test.end(), target);
std::cout << std::distance(test.begin(), it) << std::endl;
}
@ -21,17 +19,9 @@ int main() {
test_bsearch(test, 8); // 14
test_bsearch(test, -1); // 14
test_bsearch(test, 0); // 0, 1
test_bsearch(test, 0, BsearchPolicy::FIRST); // 0
test_bsearch(test, 0, BsearchPolicy::LAST); // 1
test_bsearch(test, 4); // 5, 6
test_bsearch(test, 4, BsearchPolicy::FIRST); // 5
test_bsearch(test, 4, BsearchPolicy::LAST); // 6
test_bsearch(test, 5); // 7, 8, 9, 10, 11
test_bsearch(test, 5, BsearchPolicy::FIRST); // 7
test_bsearch(test, 5, BsearchPolicy::LAST); // 11
test_bsearch(test, 7); // 13
test_bsearch(test, 7, BsearchPolicy::FIRST); // 13
test_bsearch(test, 7, BsearchPolicy::LAST); // 13
return 0;
}

0
c-cpp/16_bsearch/.gitkeep Normal file
View File

283
c-cpp/16_bsearch/bsearch_variant.c Normal file
View File

@ -0,0 +1,283 @@
#include <stdio.h>
#include <stdlib.h>
#include <stdbool.h>
#include <string.h>
int binary_search(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
if (arr[mid] == val)
return mid;
if (arr[mid] < val)
low = mid + 1;
else
high = mid - 1;
}
return -1;
}
/*
* find the first index with *val*
*
* This is a little tricky because the calculation of mid is integer based, it
* will be cast to the lower bound of an integer.
*
* In case the [low, high] range is of size 1 or 2 and arr[mid] >= val, we will
* have:
*
* mid = (low + high) / 2 = low
* high = mid - 1 = low - 1 < low, which break the loop
*
*/
int binary_search_first(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
//printf("[%d-%d] %d\n", low, high, mid);
if (arr[mid] >= val)
high = mid - 1;
else
low = mid + 1;
}
//printf("[%d-%d] %d\n", low, high, mid);
if (arr[low] == val)
return low;
else
return -1;
}
int binary_search_last(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
if (arr[mid] <= val)
low = mid + 1;
else
high = mid - 1;
}
if (arr[high] == val)
return high;
else
return -1;
}
int binary_search_first_r(int *arr, int size, int val)
{
int mid = size / 2;
int idx;
if (size <= 0)
return -1;
// we find *val* at mid, try first half
if (arr[mid] == val) {
idx = binary_search_first_r(arr, mid, val);
return idx != -1 ? idx : mid;
}
// mid == 0 means size == 1
// so the only element in array doesn't equal to val
if (!mid)
return -1;
if (arr[mid] < val) {
idx = binary_search_first_r(arr + mid + 1, size - mid - 1, val);
if (idx != -1)
idx += mid + 1;
} else {
idx = binary_search_first_r(arr, mid, val);
}
return idx;
}
int binary_search_last_r(int *arr, int size, int val)
{
int mid = size / 2;
int idx;
if (size <= 0)
return -1;
// we find *val* at mid, try last half
if (arr[mid] == val) {
idx = binary_search_last_r(arr+mid+1, size-mid-1, val);
if (idx != -1)
mid += idx + 1;
return mid;
}
// mid == 0 means size == 1
// so the only element in array doesn't equal to val
if (!mid)
return -1;
if (arr[mid] < val) {
idx = binary_search_last_r(arr + mid + 1, size - mid - 1, val);
if (idx != -1)
idx += mid + 1;
} else {
idx = binary_search_last_r(arr, mid, val);
}
return idx;
}
int binary_search_first_bigger(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
if (arr[mid] >= val) {
if (mid == 0 || arr[mid-1] < val)
return mid;
high = mid - 1;
} else {
low = mid + 1;
}
}
return -1;
}
int binary_search_first_bigger_r(int *arr, int size, int val)
{
int mid = size / 2;
int idx;
if (size <= 0)
return -1;
if (arr[mid] >= val) {
// find one bigger than val, try first half
idx = binary_search_first_bigger_r(arr, mid, val);
if (idx == -1)
idx = mid;
} else {
// the bigger one may sit in second half
idx = binary_search_first_bigger_r(arr + mid + 1, size - mid - 1, val);
if (idx != -1)
idx += mid + 1;
}
return idx;
}
int binary_search_last_smaller(int *arr, int size, int val)
{
int low = 0, high = size - 1, mid;
while (low <= high) {
mid = (low + high) / 2;
if (arr[mid] <= val) {
if (mid == 0 || arr[mid+1] > val)
return mid;
low = mid + 1;
} else {
high = mid - 1;
}
}
return -1;
}
int binary_search_last_smaller_r(int *arr, int size, int val)
{
int mid = size / 2;
int idx;
if (size <= 0)
return -1;
if (arr[mid] <= val) {
// find one smaller than val, try second half
idx = binary_search_last_smaller_r(arr + mid + 1, size - mid - 1, val);
if (idx != -1)
idx += mid + 1;
else
idx = mid;
} else {
// the smaller one may sit in first half
idx = binary_search_last_smaller_r(arr, mid, val);
}
return idx;
}
int main()
{
int arr[10] = {1, 4, 5, 9, 12, 14, 19, 19, 31, 36};
int idx;
printf("Test Array:\n");
for (idx = 0; idx < 10; idx++)
printf("%8d", arr[idx]);
printf("\n");
idx = binary_search_first(arr, 10, 19);
if (idx != -1)
printf("first 19 at %d\n", idx);
else
printf("19 not in arr \n");
idx = binary_search_first_r(arr, 10, 19);
if (idx != -1)
printf("first 19 at %d\n", idx);
else
printf("19 not in arr \n");
idx = binary_search_last(arr, 10, 19);
if (idx != -1)
printf("last 19 at %d\n", idx);
else
printf("19 not in arr \n");
idx = binary_search_last_r(arr, 10, 19);
if (idx != -1)
printf("last 19 at %d\n", idx);
else
printf("19 not in arr \n");
idx = binary_search_first_bigger(arr, 10, 12);
if (idx != -1)
printf("first bigger 12 at %d\n", idx);
else
printf("12 not in arr \n");
idx = binary_search_first_bigger_r(arr, 10, 12);
if (idx != -1)
printf("first bigger 12 at %d\n", idx);
else
printf("12 not in arr \n");
idx = binary_search_last_smaller(arr, 10, 12);
if (idx != -1)
printf("last smaller 12 at %d\n", idx);
else
printf("12 not in arr \n");
idx = binary_search_last_smaller_r(arr, 10, 12);
if (idx != -1)
printf("last smaller 12 at %d\n", idx);
else
printf("12 not in arr \n");
return 0;
}

90
c-cpp/16_bsearch/bsearch_varients.hpp Normal file
View File

@ -0,0 +1,90 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#ifndef BSEARCH_BSEARCH_VARIENTS_HPP_
#define BSEARCH_BSEARCH_VARIENTS_HPP_
#include <iterator>
#include <functional>
enum class BsearchPolicy { UNSPECIFIED, FIRST, LAST, FIRST_NOT_LESS, LAST_NOT_GREATER };
// Liam Huang: The algorithm works right with iterators that meet the ForwardIterator requirement,
// but with a bad time complexity. For better performance, iterators should meet
// the RandomAccessIterator requirement.
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare>
IterT bsearch(IterT first,
IterT last,
ValueT target,
Compare comp,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
IterT result = last;
while (std::distance(first, last) > 0) {
IterT mid = first + std::distance(first, last) / 2;
if (policy == BsearchPolicy::FIRST_NOT_LESS) {
if (!comp(*mid, target)) {
if (mid == first or comp(*(mid - 1), target)) {
result = mid;
break;
} else {
last = mid;
}
} else {
first = mid + 1;
}
} else if (policy == BsearchPolicy::LAST_NOT_GREATER) {
if (comp(target, *mid)) {
last = mid;
} else {
if (std::distance(mid, last) == 1 or comp(target, *(mid + 1))) {
result = mid;
break;
} else {
first = mid + 1;
}
}
} else { // policy == UNSPECIFIED or FIRST or LAST
if (comp(*mid, target)) {
first = mid + 1;
} else if (comp(target, *mid)) {
last = mid;
} else { // equal
if (policy == BsearchPolicy::FIRST) {
if (mid == first or comp(*(mid - 1), *mid)) {
result = mid;
break;
} else {
last = mid;
}
} else if (policy == BsearchPolicy::LAST) {
if (std::distance(mid, last) == 1 or comp(*mid, *(mid + 1))) {
result = mid;
break;
} else {
first = mid + 1;
}
} else {
result = mid;
break;
}
}
}
}
return result;
}
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare = std::less<ValueT>>
IterT bsearch(IterT first,
IterT last,
ValueT target,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
return bsearch(first, last, target, Compare(), policy);
}
#endif // BSEARCH_BSEARCH_VARIENTS_HPP_

41
c-cpp/16_bsearch/bsearch_varients_test.cc Normal file
View File

@ -0,0 +1,41 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#include <iostream>
#include <vector>
#include "bsearch_varients.hpp"
template <typename VecT, typename T = typename VecT::value_type>
void test_bsearch(const VecT& test,
T target,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
auto it = bsearch(test.begin(), test.end(), target, policy);
std::cout << std::distance(test.begin(), it) << std::endl;
}
int main() {
std::vector<int> test{0, 0, 1, 2, 3, 4, 4, 5, 5, 5, 5, 5, 6, 8}; // std::less<int>()
test_bsearch(test, 8); // 14
test_bsearch(test, -1); // 14
test_bsearch(test, 0); // 0, 1
test_bsearch(test, 0, BsearchPolicy::FIRST); // 0
test_bsearch(test, 0, BsearchPolicy::LAST); // 1
test_bsearch(test, 4); // 5, 6
test_bsearch(test, 4, BsearchPolicy::FIRST); // 5
test_bsearch(test, 4, BsearchPolicy::LAST); // 6
test_bsearch(test, 5); // 7, 8, 9, 10, 11
test_bsearch(test, 5, BsearchPolicy::FIRST); // 7
test_bsearch(test, 5, BsearchPolicy::LAST); // 11
test_bsearch(test, 7, BsearchPolicy::FIRST_NOT_LESS); // 13
test_bsearch(test, 7, BsearchPolicy::LAST_NOT_GREATER); // 12
test_bsearch(test, 7, BsearchPolicy::FIRST); // 14
test_bsearch(test, 8); // 13
test_bsearch(test, 8, BsearchPolicy::FIRST); // 13
test_bsearch(test, 8, BsearchPolicy::LAST); // 13
return 0;
}

0
c-cpp/17_skiplist/.gitkeep Normal file
View File

186
c-cpp/17_skiplist/skiplist.hpp Normal file
View File

@ -0,0 +1,186 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/29.
*/
#ifndef SKIPLIST_SKIPLIST_HPP_
#define SKIPLIST_SKIPLIST_HPP_
#include <functional>
#include <type_traits>
#include <vector>
#include <chrono>
#include <random>
#include <initializer_list>
#include <limits>
#include <iostream>
template <typename Value>
class skiplist {
public:
using value_type = Value;
using hash_type = std::hash<value_type>;
using key_type = typename hash_type::result_type;
using size_type = size_t;
private:
struct InternalNode {
value_type value;
const key_type key;
std::vector<InternalNode*> forwards; // pointers to successor nodes
InternalNode(const key_type& k, const size_type lv)
: value(), key(k), forwards(lv, nullptr) {}
InternalNode(const value_type& v, const size_type lv)
: value(v), key(hash_type()(value)), forwards(lv, nullptr) {}
};
using node_type = InternalNode;
private:
const size_type MAX_LEVEL = 16;
const double PROBABILITY = 0.5;
const unsigned int seed =
std::chrono::system_clock::now().time_since_epoch().count();
mutable
std::default_random_engine generator = std::default_random_engine(seed);
mutable
std::binomial_distribution<size_type> distribution =
std::binomial_distribution<size_type>(MAX_LEVEL - 1, PROBABILITY);
node_type* head = nullptr;
node_type* nil = nullptr;
static const value_type default_value;
public:
skiplist() {
key_type head_key = std::numeric_limits<key_type>::min();
key_type nil_key = std::numeric_limits<key_type>::max();
head = new node_type(head_key, MAX_LEVEL);
nil = new node_type(nil_key, MAX_LEVEL);
std::fill(head->forwards.begin(), head->forwards.end(), nil);
}
skiplist(std::initializer_list<value_type> init) : skiplist() {
for (const value_type& v : init) {
insert(v);
}
}
skiplist(const skiplist& other) = delete;
skiplist(skiplist&& other) :
MAX_LEVEL(std::move(other.MAX_LEVEL)),
PROBABILITY(std::move(other.PROBABILITY)),
seed(std::move(other.seed)),
generator(std::move(other.generator)),
distribution(std::move(other.distribution)),
head(other.head),
nil(other.nil) {
other.head = nullptr;
other.nil = nullptr;
}
~skiplist() {
node_type* node = head;
while (nullptr != node and nullptr != node->forwards[0]) {
node_type* tmp = node;
node = node->forwards[0];
delete tmp;
}
delete node;
}
skiplist& operator=(const skiplist& other) = delete;
skiplist& operator=(skiplist&& other) = delete;
private:
inline size_type get_random_level() const {
return distribution(generator);
}
static size_type get_node_level(const node_type* node) {
return node->forwards.size();
}
static node_type* make_node(const value_type& v, const size_type lv) {
return new node_type(v, lv);
}
/**
* @brief returns a pointer to the first node such that
* node->key == hash_type()(v) and node->value == v.
*/
node_type* get_first_equal(const value_type& v) const {
const key_type target = hash_type()(v);
node_type* x = head;
for (size_type i = get_node_level(head); i > 0; --i) {
while (x->forwards[i - 1]->key < target or
x->forwards[i - 1]->key == target and x->forwards[i - 1]->value != v) {
x = x->forwards[i - 1];
}
}
return x->forwards[0];
}
/**
* @brief returns a collection of nodes.
* returns[i] is the pointer to the last node at level i + 1
* such that returns[i]->key < hash_type()(v) or
* returns[i]->key == hash_type()(v) but returns[i]->value != v.
*/
std::vector<node_type*> get_predecessors(const value_type& v) const {
const key_type target = hash_type()(v);
std::vector<node_type*> results(get_node_level(head), nullptr);
node_type* x = head;
for (size_type i = get_node_level(head); i > 0; --i) {
while (x->forwards[i - 1]->key < target or
x->forwards[i - 1]->key == target and x->forwards[i - 1]->value != v) {
x = x->forwards[i - 1];
}
results[i - 1] = x;
}
return results;
}
public:
const value_type& find(const value_type& target) {
node_type* x = get_first_equal(target);
if (nullptr != x and nil != x and x->value == target) {
return x->value;
} else {
return default_value;
}
}
void insert(const value_type& value) {
std::vector<node_type*> preds = get_predecessors(value);
const size_type new_node_lv = get_random_level();
node_type* new_node = make_node(value, new_node_lv);
for (size_type i = 0; i != new_node_lv; ++i) {
new_node->forwards[i] = preds[i]->forwards[i];
preds[i]->forwards[i] = new_node;
}
}
void erase(const value_type& value) {
std::vector<node_type*> preds = get_predecessors(value);
node_type* node = preds[0]->forwards[0];
if (node == nil or node->value != value) { return; }
for (size_type i = 0; i != get_node_level(node); ++i) {
preds[i]->forwards[i] = node->forwards[i];
}
delete node;
}
void print(std::ostream& os) const {
node_type* list = head->forwards[0];
os << "{";
while (list != nil) {
os << "key: " << list->key << " value: " << list->value
<< " level: " << get_node_level(list);
list = list->forwards[0];
if (list != nil) os << " : ";
os << "\n";
}
os << "}\n";
}
};
template <typename Value>
const typename skiplist<Value>::value_type skiplist<Value>::default_value =
typename skiplist<Value>::value_type();
#endif // SKIPLIST_SKIPLIST_HPP_

67
c-cpp/17_skiplist/skiplist_test.cc Normal file
View File

@ -0,0 +1,67 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/30.
*/
#include <iostream>
#include <string>
#include "skiplist.hpp"
int main() {
// 1. Initialize a skip list for test
// * default constructor
// * constructor with initializer list
// * insert
skiplist<std::string> ss{"1", "2", "3", "4", "5"};
// 1a. show
// * print
ss.print(std::cout);
std::cout << std::endl;
// 2. move construction
// * move constructor
skiplist<std::string> s(std::move(ss));
// 2a. show
// * print
s.print(std::cout);
std::cout << std::endl;
// 3.a find something doesn't exist.
// * find
auto f = s.find("0");
if (!f.empty()) {
std::cout << "Node found!\nvalue: " << f << '\n';
} else {
std::cout << "Node NOT found!\n";
}
// 3.b find something does exist.
// * find
auto ff = s.find("1");
if (!ff.empty()) {
std::cout << "Node found!\tvalue: " << ff << '\n';
} else {
std::cout << "Node NOT found!\n";
}
// 4. insert() - reassign
s.insert("TEST");
// 4a. print()
s.print(std::cout);
std::cout << std::endl;
// 5. erase()
s.erase("TEST");
// 5a. print();
s.print(std::cout);
std::cout << std::endl;
std::cout << "\nDone!\n";
return 0;
// 6. destructor
}

366
c-cpp/17_skiplist/skiplist_tr.hpp Normal file
View File

@ -0,0 +1,366 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/30.
*/
#ifndef SKIPLIST_SKIPLIST_TR_HPP_
#define SKIPLIST_SKIPLIST_TR_HPP_
#ifdef LIAM_UT_DEBUG_
#include <assert.h>
#include <iostream>
#endif
#include <set>
#include <vector>
#include <list>
#include <functional>
#include <type_traits>
#include <random>
#include <limits>
#include <algorithm>
#include <initializer_list>
#include <iterator>
namespace skiplist_detail {
template <typename Key, typename Value>
struct InternalNode {
using iterator = typename std::list<InternalNode>::iterator;
const Key key;
std::multiset<Value> values;
std::vector<iterator> forwards;
InternalNode() = delete;
explicit InternalNode(const Key& k) : key(k) {}
};
template <typename IntType>
class random_level {
private:
mutable std::random_device rd;
mutable std::mt19937 gen = std::mt19937(rd());
mutable std::binomial_distribution<IntType> dist;
public:
random_level(IntType max_level, double prob) : dist(max_level - 1, prob) {}
inline IntType operator()() const { return dist(gen); }
};
} // namespace skiplist_detail
enum class erase_policy { ALL, SINGLE };
template <typename Value,
typename Hash = std::hash<Value>,
size_t Factor = 2>
class skiplist {
public:
using value_type = Value;
using size_type = size_t;
using hasher = Hash;
using hash_type = typename Hash::result_type;
using compare = std::less<hash_type>;
using node_type = skiplist_detail::InternalNode<hash_type, value_type>;
using container = std::list<node_type>;
using iterator = typename container::iterator;
using const_iterator = typename container::const_iterator;
static_assert(std::is_same<iterator, typename node_type::iterator>::value,
"STATIC ASSERT FAILED! iterator type differs.");
private:
size_type max_lv_ = 2;
double prob_ = 0.5;
mutable skiplist_detail::random_level<size_type> rl_;
container cont_;
public:
skiplist() : rl_(max_lv_, prob_) {
init_internally();
}
explicit skiplist(const size_type max_lv, const double prob = 0.5)
: max_lv_(max_lv), prob_(prob), rl_(max_lv_, prob_) {
init_internally();
}
skiplist(skiplist&& other) = default;
skiplist& operator=(skiplist&& other) = default;
~skiplist() = default;
template <typename InputIt>
skiplist(InputIt first, InputIt last) : skiplist() {
using value_type_in_iter = typename std::iterator_traits<InputIt>::value_type;
static_assert(std::is_same<value_type, value_type_in_iter>::value,
"STATIC ASSERT FAILED! Value in InputIt should be the same to value_type.");
for (InputIt i = first; i != last; ++i) {
insert(*i);
}
}
skiplist(std::initializer_list<value_type> init) : skiplist(init.begin(), init.end()) {}
private: // noncopyable
skiplist(const skiplist&) = delete;
skiplist& operator=(const skiplist&) = delete;
private:
void init_internally() {
const hash_type tail_key = std::numeric_limits<hash_type>::max();
node_type tail(tail_key);
tail.forwards.resize(max_lv_);
std::fill(tail.forwards.begin(), tail.forwards.end(), cont_.end());
cont_.insert(cont_.begin(), std::move(tail));
const hash_type head_key = std::numeric_limits<hash_type>::min();
node_type head(head_key);
head.forwards.resize(max_lv_);
cont_.insert(cont_.begin(), std::move(head));
std::fill(cont_.begin()->forwards.begin(), cont_.begin()->forwards.end(),
std::next(cont_.begin()));
#ifdef LIAM_UT_DEBUG_
assert(cont_.begin()->key == head_key);
for (auto it : cont_.begin()->forwards) {
assert(it->key == tail_key);
}
for (auto it : std::next(cont_.begin())->forwards) {
assert(it == cont_.end());
}
std::cerr << "UT_DEBUG: all assert in init_internally() success!\n";
#endif
return;
}
/**
* @brief return a const_iterator points to the last element
* such that its hash_key <= target_hash_key
*/
const_iterator find_helper(const hash_type& key) const {
#ifdef LIAM_UT_DEBUG_
std::cerr << "Keys contained in the list: ";
for (auto node : cont_) {
std::cerr << node.key << ' ';
}
std::cerr << '\n';
std::cerr << "Target key: " << key << '\n';
#endif
const_iterator iter = begin();
for (size_type i = 0; i != max_lv_; ++i) {
size_type focus = max_lv_ - 1 - i;
// invariant: iter->key <= key
while (not compare()(key, iter->forwards[focus]->key)) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "i: " << i << " focus: " << focus << ". "
<< "since iter->forwards[focus]->key[" << iter->forwards[focus]->key
<< "] <= key[" << key << "], ";
#endif
iter = iter->forwards[focus];
#ifdef LIAM_UT_DEBUG_
std::cerr << "step forward iter to [" << iter->key << "]\n";
#endif
}
// result: iter->key <= key < iter->forwards[focus]->key
#ifdef LIAM_UT_DEBUG_
std::cerr << "The following fact holds at level " << focus
<< ": iter->key[" << iter->key << "] <= key["
<< key << "] < iter->forwards[focus]->key[" << iter->forwards[focus]->key
<<"].\n";
#endif
}
return iter;
}
std::vector<iterator> find_predecessors(const hash_type& key, const size_type& lv) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "Keys contained in the list: ";
for (auto node : cont_) {
std::cerr << node.key << ' ';
}
std::cerr << '\n';
std::cerr << "Target key: " << key << '\n';
#endif
std::vector<iterator> res;
res.resize(lv + 1);
iterator iter = begin();
for (size_type i = 0; i != max_lv_; ++i) {
size_type focus = max_lv_ - 1 - i;
#ifdef LIAM_UT_DEBUG_
std::cerr << "i: " << i << " focus: " << focus << ".\n";
#endif
// invariant: iter->key < key
while (compare()(iter->forwards[focus]->key, key)) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "since iter->forwards[focus]->key[" << iter->forwards[focus]->key
<< "] < key[" << key << "], ";
#endif
iter = iter->forwards[focus];
#ifdef LIAM_UT_DEBUG_
std::cerr << "step forward iter to [" << iter->key << "]\n";
#endif
}
// result: iter->key < key <= iter->forwards[focus]->key
#ifdef LIAM_UT_DEBUG_
std::cerr << "The following fact holds at level " << focus
<< ": iter->key[" << iter->key << "] < key[" << key
<< "] <= iter->forwards[focus]->key[" << iter->forwards[focus]->key
<<"].\n";
#endif
if (focus < lv + 1) {
res[focus] = iter;
#ifdef LIAM_UT_DEBUG_
std::cerr << "predecessor at level [" << focus
<< "] has been recorded, while level upper limit is " << lv <<".\n";
#endif
}
}
return res;
}
public:
size_type size() const {
return cont_.size() - 2;
}
bool empty() const {
return size() == 0;
}
iterator begin() {
return cont_.begin();
}
const_iterator begin() const {
return cont_.cbegin();
}
const_iterator cbegin() const {
return cont_.cbegin();
}
iterator end() {
return cont_.end();
}
const_iterator end() const {
return cont_.cend();
}
const_iterator cend() const {
return cont_.cend();
}
void grow(const size_type new_max_lv) {
if (max_lv_ < new_max_lv) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "grow from [" << max_lv_ << "] to ["
<< new_max_lv << "]!\n";
#endif
max_lv_ = new_max_lv;
iterator tail = std::prev(cont_.end());
auto beg_tail = tail->forwards.end();
tail->forwards.resize(max_lv_, cont_.end());
iterator head = cont_.begin();
auto beg_head = head->forwards.end();
head->forwards.resize(max_lv_, tail);
return;
} else {
#ifdef LIAM_UT_DEBUG_
std::cerr << "abandon growing!\n";
#endif
return;
}
}
void grow() {
grow(Factor * max_lv_);
}
size_type capability() const {
return std::pow(Factor, max_lv_);
}
public:
const_iterator find(const value_type& target) const {
#ifdef LIAM_UT_DEBUG_
std::cerr << "finding [" << target << "]!\n";
#endif
const hash_type key = hasher()(target);
const_iterator iter = find_helper(key);
return (iter->key == key) ? iter : cont_.end();
}
void insert(const value_type& target) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "inserting [" << target << "]!\n";
#endif
if (size() > static_cast<double>(Factor - 1) / Factor * capability()) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "size[" << size() << "], Factor[" << Factor << "], capability[" << capability() << "]!\n";
#endif
grow();
}
const hash_type key = hasher()(target);
const size_type lv = rl_();
std::vector<iterator> predecessors = find_predecessors(key, lv);
if (predecessors[0]->forwards[0]->key == key) { // key already in skiplist
#ifdef LIAM_UT_DEBUG_
std::cerr << "key [" << key << "] already in the skiplist, insert directly!\n";
#endif
predecessors[0]->forwards[0]->values.insert(target);
return;
} else {
#ifdef LIAM_UT_DEBUG_
std::cerr << "key [" << key << "] not in the skiplist, insert a new node!\n";
#endif
node_type node(key);
node.forwards.resize(lv + 1);
node.values.insert(target);
iterator inserted = cont_.insert(predecessors[0]->forwards[0], std::move(node));
for (size_type i = 0; i != lv + 1; ++i) {
inserted->forwards[i] = predecessors[i]->forwards[i];
predecessors[i]->forwards[i] = inserted;
}
#ifdef LIAM_UT_DEBUG_
assert(inserted->forwards[0] == std::next(inserted));
#endif
return;
}
}
void erase(const value_type& target,
const erase_policy policy = erase_policy::ALL) {
#ifdef LIAM_UT_DEBUG_
std::cerr << "erasing [" << target << "]!\n";
#endif
const hash_type key = hasher()(target);
std::vector<iterator> predecessors = find_predecessors(key, max_lv_);
if (predecessors[0]->forwards[0]->key == key) { // hit
#ifdef LIAM_UT_DEBUG_
std::cerr << "key [" << key << "] is in the skiplist!\n";
#endif
iterator found = predecessors[0]->forwards[0];
for (auto iter = found->values.begin(); iter != found->values.end(); ) {
if (policy == erase_policy::ALL) {
if (*iter == target) {
iter = found->values.erase(iter);
} else {
++iter;
}
} else if (policy == erase_policy::SINGLE) {
if (*iter == target) {
found->values.erase(iter);
break;
}
}
}
#ifdef LIAM_UT_DEBUG_
std::cerr << "target(s) removed!\n";
#endif
if (found->values.empty()) {
const size_type lvp1 = found->forwards.size(); // lv plus 1
for (size_type i = 0; i != lvp1; ++i) {
predecessors[i]->forwards[i] = found->forwards[i];
}
cont_.erase(found);
#ifdef LIAM_UT_DEBUG_
std::cerr << "empty node removed!\n";
#endif
return;
} else {
return;
}
} else {
#ifdef LIAM_UT_DEBUG_
std::cerr << "key [" << key << "] is not in the skiplist, do nothing!\n";
#endif
return;
}
}
};
#endif // SKIPLIST_SKIPLIST_TR_HPP_

107
c-cpp/17_skiplist/skiplist_tr_test.cc Normal file
View File

@ -0,0 +1,107 @@
/**
* Created by Liam Huang (Liam0205) on 2018/10/30.
*/
#include <assert.h>
#include <iostream>
#include <map>
#include <string>
#include "skiplist_tr.hpp"
int main() {
// 1. UT for skiplist_detail::random_level
skiplist_detail::random_level<size_t> rl(5, 0.5);
std::map<size_t, size_t> hist;
for (size_t i = 0; i != 10000; ++i) {
++hist[rl()];
}
for (auto p : hist) {
std::cout << p.first << ' ' << std::string(p.second / 100, '*') << '\n';
}
// 2. UT for skiplist(), init_internally(), size(), empty()
skiplist<std::string> sl_default;
assert(sl_default.empty());
// 2.1. UT for grow with abandon
sl_default.grow(1);
assert(sl_default.capability() == 4);
// 2.2. UT for grow
sl_default.grow(10);
assert(sl_default.capability() == 1024);
// 3. UT for constructor of initializer_list and InputIt, init_internally(), insert(),
// find_predecessors()
skiplist<std::string> sl{"hello", "world", "!"};
assert(not sl.empty());
assert(3 == sl.size());
// 4. UT for find() find_helper()
auto search = sl_default.find("nonexist");
assert(search == sl_default.cend());
assert(search == sl_default.end());
search = sl.find("hello");
assert(search != sl.cend());
assert(search != sl.end());
search = sl.find("nonexist");
assert(search == sl.cend());
assert(search == sl.end());
// 5. UT for insert(), find_predecessors()
// 5.1. UT for insert a already-exist item
sl.insert("hello");
search = sl.find("hello");
assert(search != sl.cend());
assert(search != sl.end());
// 5.2. UT for insert a new incoming item
search = sl.find("now exist");
assert(search == sl.cend());
assert(search == sl.end());
sl.insert("now exist");
search = sl.find("now exist");
assert(search != sl.cend());
assert(search != sl.end());
// 6. UT for erase(), find_predecessors()
sl.insert("hello");
sl.insert("hello");
sl.insert("hello");
sl.insert("hello");
// 6.1. UT for erase single item
sl.erase("hello", erase_policy::SINGLE);
search = sl.find("hello");
assert(search != sl.cend());
assert(search != sl.end());
// 6.2. UT for erase all items
sl.erase("hello", erase_policy::ALL);
search = sl.find("hello");
assert(search == sl.cend());
assert(search == sl.end());
// 6.3 UT for erase non-exist item
sl.erase("nonexist");
// 7. UT for insert() behind erase()
// 7.1. different word
sl.insert("world");
search = sl.find("world");
assert(search != sl.cend());
assert(search != sl.end());
assert(search->values.count("world") == 2);
// 7.1. same word, also UT for grow()
search = sl.find("hello");
assert(search == sl.cend());
assert(search == sl.end());
sl.insert("hello");
sl.insert("hello");
sl.insert("hello");
sl.insert("hello");
sl.insert("hello");
search = sl.find("hello");
assert(search != sl.cend());
assert(search != sl.end());
assert(search->values.count("hello") == 5);
return 0;
// 8. UT for ~skiplist()
}

63
f21 Normal file
View File

@ -0,0 +1,63 @@
// A Stack based C++ program to find next
// greater element for all array elements
// in same order as input.
#include <bits/stdc++.h>
using namespace std;
/* prints element and NGE pair for all
elements of arr[] of size n */
void printNGE(int arr[], int n)
{
stack<int> s;
unordered_map<int, int> mp;
/* push the first element to stack */
s.push(arr[0]);
// iterate for rest of the elements
for (int i = 1; i < n; i++) {
if (s.empty()) {
s.push(arr[i]);
continue;
}
/* if stack is not empty, then
pop an element from stack.
If the popped element is smaller
than next, then
a) print the pair
b) keep popping while elements are
smaller and stack is not empty */
while (s.empty() == false && s.top() < arr[i]) {
mp[s.top()] = arr[i];
s.pop();
}
/* push next to stack so that we can find
next smaller for it */
s.push(arr[i]);
}
/* After iterating over the loop, the remaining
elements in stack do not have the next smaller
element, so print -1 for them */
while (s.empty() == false) {
mp[s.top()] = -1;
s.pop();
}
for (int i=0; i<n; i++)
cout << arr[i] << " ---> " << mp[arr[i]] << endl;
}
/* Driver program to test above functions */
int main()
{
int arr[] = { 11, 13, 21, 3 };
int n = sizeof(arr) / sizeof(arr[0]);
printNGE(arr, n);
return 0;
}

108
go/15_binarysearch/binarysearch.go
View File

@ -45,3 +45,111 @@ func bs(a []int, v int, low, high int) int {
return bs(a, v, mid+1, high)
}
}
//查找第一个等于给定值的元素
func BinarySearchFirst(a []int, v int) int {
n := len(a)
if n == 0 {
return -1
}
low := 0
high := n - 1
for low <= high {
mid := (low + high) >> 1
if a[mid] > v {
high = mid - 1
} else if a[mid] < v {
low = mid + 1
} else {
if mid == 0 || a[mid-1] != v {
return mid
} else {
high = mid - 1
}
}
}
return -1
}
//查找最后一个值等于给定值的元素
func BinarySearchLast(a []int, v int) int {
n := len(a)
if n == 0 {
return -1
}
low := 0
high := n - 1
for low <= high {
mid := (low + high) >> 1
if a[mid] > v {
high = mid - 1
} else if a[mid] < v {
low = mid + 1
} else {
if mid == n-1 || a[mid+1] != v {
return mid
} else {
low = mid + 1
}
}
}
return -1
}
//查找第一个大于等于给定值的元素
func BinarySearchFirstGT(a []int, v int) int {
n := len(a)
if n == 0 {
return -1
}
low := 0
high := n - 1
for low <= high {
mid := (high + low) >> 1
if a[mid] > v {
high = mid - 1
} else if a[mid] < v {
low = mid + 1
} else {
if mid != n-1 && a[mid+1] > v {
return mid + 1
} else {
low = mid + 1
}
}
}
return -1
}
//查找最后一个小于等于给定值的元素
func BinarySearchLastLT(a []int, v int) int {
n := len(a)
if n == 0 {
return -1
}
low := 0
high := n - 1
for low <= high {
mid := (low + high) >> 1
if a[mid] > v {
high = mid - 1
} else if a[mid] < v {
low = mid + 1
} else {
if mid == 0 || a[mid-1] < v {
return mid - 1
} else {
high = mid - 1
}
}
}
return -1
}

76
go/15_binarysearch/binarysearch_test.go
View File

@ -25,3 +25,79 @@ func TestBinarySearchRecursive(t *testing.T) {
t.Fatal(BinarySearch(a, 4))
}
}
func TestBinarySearchFirst(t *testing.T) {
var a []int
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirst(a, 2) != 1 {
t.Fatal(BinarySearchFirst(a, 2))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirst(a, 3) != 4 {
t.Fatal(BinarySearchFirst(a, 3))
}
}
func TestBinarySearchLast(t *testing.T) {
var a []int
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLast(a, 2) != 3 {
t.Fatal(BinarySearchLast(a, 2))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLast(a, 3) != 4 {
t.Fatal(BinarySearchLast(a, 3))
}
}
func TestBinarySearchFirstGT(t *testing.T) {
var a []int
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirstGT(a, 2) != 4 {
t.Fatal(BinarySearchFirstGT(a, 2))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirstGT(a, 1) != 1 {
t.Fatal(BinarySearchFirstGT(a, 1))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirstGT(a, 3) != 5 {
t.Fatal(BinarySearchFirstGT(a, 3))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchFirstGT(a, 4) != -1 {
t.Fatal(BinarySearchFirstGT(a, 4))
}
}
func TestBinarySearchLastLT(t *testing.T) {
var a []int
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLastLT(a, 2) != 0 {
t.Fatal(BinarySearchLastLT(a, 2))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLastLT(a, 1) != -1 {
t.Fatal(BinarySearchLastLT(a, 1))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLastLT(a, 3) != 3 {
t.Fatal(BinarySearchLastLT(a, 3))
}
a = []int{1, 2, 2, 2, 3, 4}
if BinarySearchLastLT(a, 4) != 4 {
t.Fatal(BinarySearchLastLT(a, 4))
}
}

177
go/17_skiplist/skiplist.go Normal file
View File

@ -0,0 +1,177 @@
package _7_skiplist
import (
"fmt"
"math"
"math/rand"
)
const (
//最高层数
MAX_LEVEL = 16
)
//跳表节点结构体
type skipListNode struct {
//跳表保存的值
v interface{}
//用于排序的分值
score int
//层高
level int
//每层前进指针
forwards []*skipListNode
}
//新建跳表节点
func newSkipListNode(v interface{}, score, level int) *skipListNode {
return &skipListNode{v: v, score: score, forwards: make([]*skipListNode, level, level), level: level}
}
//跳表结构体
type SkipList struct {
//跳表头结点
head *skipListNode
//跳表当前层数
level int
//跳表长度
length int
}
//实例化跳表对象
func NewSkipList() *SkipList {
//头结点，便于操作
head := newSkipListNode(0, math.MinInt32, MAX_LEVEL)
return &SkipList{head, 1, 0}
}
//获取跳表长度
func (sl *SkipList) Length() int {
return sl.length
}
//获取跳表层级
func (sl *SkipList) Level() int {
return sl.level
}
//插入节点到跳表中
func (sl *SkipList) Insert(v interface{}, score int) int {
if nil == v {
return 1
}
//查找插入位置
cur := sl.head
//记录每层的路径
update := [MAX_LEVEL]*skipListNode{}
i := MAX_LEVEL - 1
for ; i >= 0; i-- {
for nil != cur.forwards[i] {
if cur.forwards[i].v == v {
return 2
}
if cur.forwards[i].score > score {
update[i] = cur
break
}
cur = cur.forwards[i]
}
if nil == cur.forwards[i] {
update[i] = cur
}
}
//通过随机算法获取该节点层数
level := 1
for i := 1; i < MAX_LEVEL; i++ {
if rand.Int31()%7 == 1 {
level++
}
}
//创建一个新的跳表节点
newNode := newSkipListNode(v, score, level)
//原有节点连接
for i := 0; i <= level-1; i++ {
next := update[i].forwards[i]
update[i].forwards[i] = newNode
newNode.forwards[i] = next
}
//如果当前节点的层数大于之前跳表的层数
//更新当前跳表层数
if level > sl.level {
sl.level = level
}
//更新跳表长度
sl.length++
return 0
}
//查找
func (sl *SkipList) Find(v interface{}, score int) *skipListNode {
if nil == v || sl.length == 0 {
return nil
}
cur := sl.head
for i := sl.level - 1; i >= 0; i-- {
for nil != cur.forwards[i] {
if cur.forwards[i].score == score && cur.forwards[i].v == v {
return cur.forwards[i]
} else if cur.forwards[i].score > score {
break
}
cur = cur.forwards[i]
}
}
return nil
}
//删除节点
func (sl *SkipList) Delete(v interface{}, score int) int {
if nil == v {
return 1
}
//查找前驱节点
cur := sl.head
//记录前驱路径
update := [MAX_LEVEL]*skipListNode{}
for i := sl.level - 1; i >= 0; i-- {
update[i] = sl.head
for nil != cur.forwards[i] {
if cur.forwards[i].score == score && cur.forwards[i].v == v {
update[i] = cur
break
}
cur = cur.forwards[i]
}
}
cur = update[0].forwards[0]
for i := cur.level - 1; i >= 0; i-- {
if update[i] == sl.head && cur.forwards[i] == nil {
sl.level = i
}
if nil == update[i].forwards[i] {
update[i].forwards[i] = nil
} else {
update[i].forwards[i] = update[i].forwards[i].forwards[i]
}
}
sl.length--
return 0
}
func (sl *SkipList) String() string {
return fmt.Sprintf("level:%+v, length:%+v", sl.level, sl.length)
}

38
go/17_skiplist/skiplist_test.go Normal file
View File

@ -0,0 +1,38 @@
package _7_skiplist
import "testing"
func TestSkipList(t *testing.T) {
sl := NewSkipList()
sl.Insert("leo", 95)
t.Log(sl.head.forwards[0])
t.Log(sl.head.forwards[0].forwards[0])
t.Log(sl)
t.Log("-----------------------------")
sl.Insert("jack", 88)
t.Log(sl.head.forwards[0])
t.Log(sl.head.forwards[0].forwards[0])
t.Log(sl.head.forwards[0].forwards[0].forwards[0])
t.Log(sl)
t.Log("-----------------------------")
sl.Insert("lily", 100)
t.Log(sl.head.forwards[0])
t.Log(sl.head.forwards[0].forwards[0])
t.Log(sl.head.forwards[0].forwards[0].forwards[0])
t.Log(sl.head.forwards[0].forwards[0].forwards[0].forwards[0])
t.Log(sl)
t.Log("-----------------------------")
t.Log(sl.Find("jack", 88))
t.Log("-----------------------------")
sl.Delete("leo", 95)
t.Log(sl.head.forwards[0])
t.Log(sl.head.forwards[0].forwards[0])
t.Log(sl.head.forwards[0].forwards[0].forwards[0])
t.Log(sl)
t.Log("-----------------------------")
}

90
go/binarysearch2.go Normal file
View File

@ -0,0 +1,90 @@
package binarySearch
//查找第一个值等于给定值得元素
func BinarySearch2(nums []int, value int) int {
length := len(nums)
start := 0
end := length - 1
for start <= end {
mid := (start + end) / 2
if nums[mid] > value {
end = mid - 1
} else if nums[mid] < value {
start = mid + 1
} else {
for mid >= 0 {
if nums[mid-1] == value {
mid--
} else {
return mid
}
}
}
}
return -1
}
// 查找最后一个值等于给定值的元素
func BinarySearch3(nums []int, value int) int {
start := 0
end := len(nums) - 1
for start <= end {
mid := (start + end) / 2
if nums[mid] > value {
end = mid - 1
} else if nums[mid] < value {
start = mid + 1
} else {
for mid < len(nums) {
if nums[mid+1] == value {
mid++
} else {
return mid
}
}
}
}
return -1
}
// 查找第一个大于等于给定值的元素
func BinarySearch4(nums []int, value int) int {
start := 0
end := len(nums) - 1
for start <= end {
mid := (start + end) >> 1
if nums[mid] < value {
start = mid + 1
} else {
for mid >= 0 {
if nums[mid-1] >= value {
mid--
} else {
return mid
}
}
}
}
return -1
}
// 查找最后一个小于等于给定值的元素
func BinarySearch5(nums []int, value int) int {
start := 0
end := len(nums) - 1
for start <= end {
mid := (start + end) >> 1
if nums[mid] > value {
end = mid - 1
} else {
for mid < len(nums) {
if nums[mid+1] <= value {
mid++
} else {
return mid
}
}
}
}
return -1
}

119
java/17_skiplist/SkipList.java Normal file
View File

@ -0,0 +1,119 @@
package skiplist;
import java.util.Random;
/**
* 跳表的一种实现方法。
* 跳表中存储的是正整数，并且存储的是不重复的。
*
* Author：ZHENG
*/
public class SkipList {
private static final int MAX_LEVEL = 16;
private int levelCount = 1;
private Node head = new Node(); // 带头链表
private Random r = new Random();
public Node find(int value) {
Node p = head;
for (int i = levelCount - 1; i >= 0; --i) {
while (p.forwards[i] != null && p.forwards[i].data < value) {
p = p.forwards[i];
}
}
if (p.forwards[0] != null && p.forwards[0].data == value) {
return p.forwards[0];
} else {
return null;
}
}
public void insert(int value) {
int level = randomLevel();
Node newNode = new Node();
newNode.data = value;
newNode.maxLevel = level;
Node update[] = new Node[level];
for (int i = 0; i < level; ++i) {
update[i] = head;
}
Node p = head;
for (int i = level - 1; i >= 0; --i) {
while (p.forwards[i] != null && p.forwards[i].data < value) {
p = p.forwards[i];
}
update[i] = p;
}
for (int i = 0; i < level; ++i) {
newNode.forwards[i] = update[i].forwards[i];
update[i].forwards[i] = newNode;
}
if (levelCount < level) levelCount = level;
}
public void delete(int value) {
Node[] update = new Node[levelCount];
Node p = head;
for (int i = levelCount - 1; i >= 0; --i) {
while (p.forwards[i] != null && p.forwards[i].data < value) {
p = p.forwards[i];
}
update[i] = p;
}
if (p.forwards[0] != null && p.forwards[0].data == value) {
for (int i = levelCount - 1; i >= 0; --i) {
if (update[i].forwards[i] != null && update[i].forwards[i].data == value) {
update[i].forwards[i] = update[i].forwards[i].forwards[i];
}
}
}
}
private int randomLevel() {
int level = 1;
for (int i = 1; i < MAX_LEVEL; ++i) {
if (r.nextInt() % 2 == 1) {
level++;
}
}
return level;
}
public void printAll() {
Node p = head;
while (p.forwards[0] != null) {
System.out.print(p.forwards[0] + " ");
p = p.forwards[0];
}
System.out.println();
}
public class Node {
private int data = -1;
private Node forwards[] = new Node[MAX_LEVEL];
private int maxLevel = 0;
@Override
public String toString() {
StringBuilder builder = new StringBuilder();
builder.append("{ data: ");
builder.append(data);
builder.append("; levels: ");
builder.append(maxLevel);
builder.append(" }");
return builder.toString();
}
}
}

27
javascript/15_binary/binaryFind.js Normal file
View File

@ -0,0 +1,27 @@
/**
* 二分查找
*
* Author: nameczz
*/
// 数组必须有序 不存在重复
const biaryFind = (sortedArr, target) => {
if (sortedArr.length === 0) return -1
let low = 0
let high = sortedArr.length - 1
while (low <= high) {
const mid = Math.floor((low + high) / 2)
if (target === sortedArr[mid]) {
return mid
} else if (target < sortedArr[mid]) {
high = mid - 1
} else {
low = mid + 1
}
}
return -1
}
const arr = [1, 4, 5, 6, 7, 8, 10, 11, 23, 42, 44, 54, 56, 77, 102]
console.log(biaryFind(arr, 44))
console.log(biaryFind(arr, 1))
console.log(biaryFind(arr, 102))
console.log(biaryFind(arr, 1111))

90
javascript/16_binary/binary-find.js Normal file
View File

@ -0,0 +1,90 @@
/**
* 二分查找
*
* Author: nameczz
*/
// 查找第一个等于给定值
const biaryFindFirst = (sortedArr, target) => {
if (sortedArr.length === 0) return -1
let low = 0
let high = sortedArr.length - 1
while (low <= high) {
const mid = Math.floor((low + high) / 2)
if (target < sortedArr[mid]) {
high = mid - 1
} else if (target > sortedArr[mid]) {
low = mid + 1
} else {
if (mid === 0 || sortedArr[mid - 1] < target) return mid
high = mid - 1
}
}
return -1
}
// 查找最后一个相等的数
const biaryFindLast = (sortedArr, target) => {
if (sortedArr.length === 0) return -1
let low = 0
let high = sortedArr.length - 1
while (low <= high) {
const mid = Math.floor((low + high) / 2)
if (target < sortedArr[mid]) {
high = mid - 1
} else if (target > sortedArr[mid]) {
low = mid + 1
} else {
if (mid === sortedArr.length - 1 || sortedArr[mid + 1] > target) return mid
low = mid + 1
}
}
return -1
}
// 查找第一个大于等于给定值的元素
const biaryFindFistBig = (sortedArr, target) => {
if (sortedArr.length === 0) return -1
let low = 0
let high = sortedArr.length - 1
while (low <= high) {
const mid = Math.floor((low + high) / 2)
if (target <= sortedArr[mid]) {
if (mid === 0 || sortedArr[mid - 1] < target) return mid
high = mid - 1
} else {
low = mid + 1
}
}
return -1
}
// 查找最后一个小于等于给定值的元素
const biaryFindLastSmall = (sortedArr, target) => {
if (sortedArr.length === 0) return -1
let low = 0
let high = sortedArr.length - 1
while (low <= high) {
const mid = Math.floor((low + high) / 2)
if (sortedArr[mid] > target) {
high = mid - 1
} else {
if (mid === sortedArr.length - 1 || sortedArr[mid + 1] > target) return mid
low = mid + 1
}
}
return -1
}
const arr = [1, 2, 3, 4, 4, 4, 4, 4, 6, 7, 8, 8, 9]
const first = biaryFindFirst(arr, 4)
console.log(`FindFirst: ${first}`)
const last = biaryFindLast(arr, 4)
console.log(`FindLast: ${last}`)
const FisrtBig = biaryFindFistBig(arr, 5)
console.log(`FindFisrtBig: ${FisrtBig}`)
const LastSmall = biaryFindLastSmall(arr, 4)
console.log(`FindLastSmall: ${LastSmall}`)

2
notes/11_sorts/readme.md
View File

@ -1,4 +1,4 @@
# 排序（上）
# 排序（平方时间复杂度排序算法）
| 排序算法 | 时间复杂度 | 是否基于比较 |
|---------|----|----|

0
notes/12_sorts/.gitkeep Normal file
View File

176
notes/12_sorts/readme.md Normal file
View File

@ -0,0 +1,176 @@
# 排序（线性对数时间复杂度排序算法）
开篇问题：如何在 $O(n)$ 时间复杂度内寻找一个无序数组中第 K 大的元素？
## 归并排序
* 归并排序使用了「分治」思想（Divide and Conquer）
* 分：把数组分成前后两部分，分别排序
* 合：将有序的两部分合并
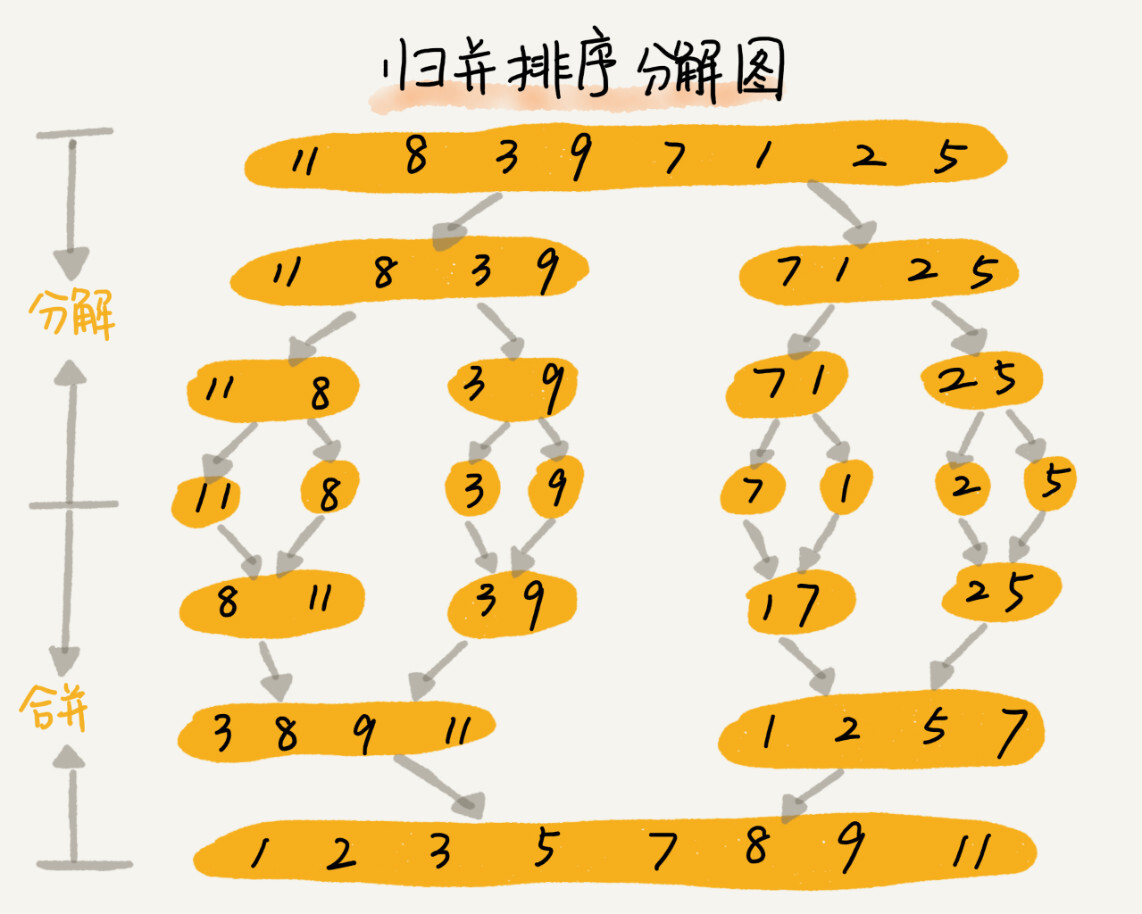
![归并排序分解图](https://static001.geekbang.org/resource/image/db/2b/db7f892d3355ef74da9cd64aa926dc2b.jpg)
* 分治与递归
* 分治：解决问题的处理办法
* 递归：实现算法的手段
* ——分治算法经常用递归来实现
* 递归实现：
* 终止条件：区间 `[first, last)` 内不足 2 个元素
* 递归公式：`merge_sort(first, last) = merge(merge_sort(first, mid), merge_sort(mid, last))`，其中 `mid = first + (last - first) / 2`
C++ 实现：
```cpp
template <typename FrwdIt,
typename T = typename std::iterator_traits<FrwdIt>::value_type,
typename BinaryPred = std::less<T>>
void merge_sort(FrwdIt first, FrwdIt last, BinaryPred comp = BinaryPred()) {
const auto len = std::distance(first, last);
if (len <= 1) { return; }
auto cut = first + len / 2;
merge_sort(first, cut, comp);
merge_sort(cut, last, comp);
std::vector<T> tmp;
tmp.reserve(len);
detail::merge(first, cut, cut, last, std::back_inserter(tmp), comp);
std::copy(tmp.begin(), tmp.end(), first);
}
```
这里涉及到一个 `merge` 的过程，它的实现大致是：
```cpp
namespace detail {
template <typename InputIt1, typename InputIt2, typename OutputIt,
typename BinaryPred = std::less<typename std::iterator_traits<InputIt1>::value_type>>
OutputIt merge(InputIt1 first1, InputIt1 last1,
InputIt2 first2, InputIt2 last2,
OutputIt d_first,
BinaryPred comp = BinaryPred()) {
for (; first1 != last1; ++d_first) {
if (first2 == last2) {
return std::copy(first1, last1, d_first);
}
if (comp(*first2, *first1)) {
*d_first = *first2;
++first2;
} else {
*d_first = *first1;
++first1;
}
}
return std::copy(first2, last2, d_first);
}
} // namespace detail
```
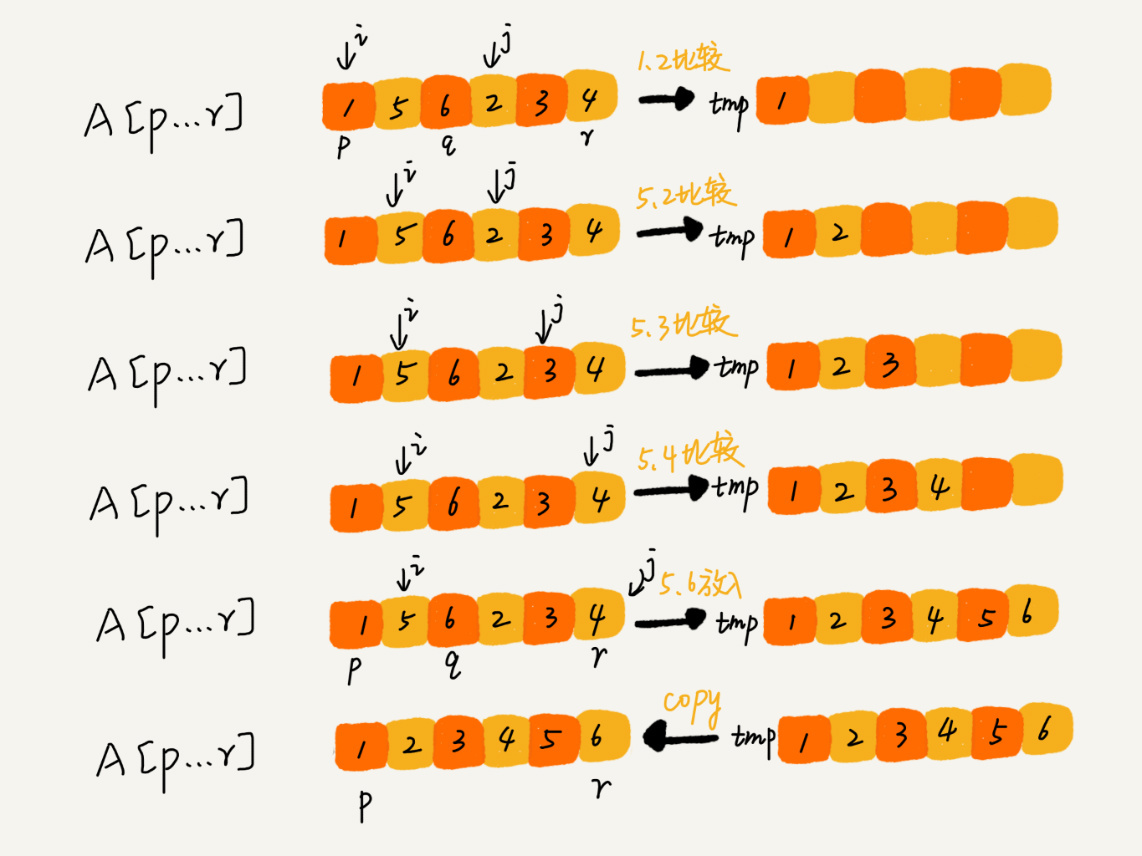
![`merge` 的过程](https://static001.geekbang.org/resource/image/95/2f/95897ade4f7ad5d10af057b1d144a22f.jpg)
### 算法分析
* 稳定性
* 由于 `comp` 是严格偏序，所以 `!comp(*first2, *first1)` 时，取用 `first1` 的元素放入 `d_first` 保证了算法稳定性
* 时间复杂度
* 定义 $T(n)$ 表示问题规模为 $n$ 时算法的耗时，
* 有递推公式：$T(n) = 2T(n/2) + n$
* 展开得 $T(n) = 2^{k}T(1) + k * n$
* 考虑 $k$ 是递归深度，它的值是 $\log_2 n$，因此 $T(n) = n + n\log_2 n$
* 因此，归并排序的时间复杂度为 $\Theta(n\log n)$
* 空间复杂度
* 一般来说，空间复杂度是 $\Theta(n)$
## 快速排序（quick sort，快排）
原理：
* 在待排序区间 `[first, last)` 中选取一个元素，称为主元（pivot，枢轴）
* 对待排序区间进行划分，使得 `[first, cut)` 中的元素满足 `comp(element, pivot)` 而 `[cut, last)` 中的元素不满足 `comp(element, pivot)`
* 对划分的两个区间，继续划分，直到区间 `[first, last)` 内不足 2 个元素
![快排分区示例](https://static001.geekbang.org/resource/image/4d/81/4d892c3a2e08a17f16097d07ea088a81.jpg)
显然，这又是一个递归：
* 终止条件：区间 `[first, last)` 内不足 2 个元素
* 递归公式：`quick_sort(first, last) = quick_sort(first, cut) + quick_sort(cut, last)`
```cpp
template <typename IterT, typename T = typename std::iterator_traits<IterT>::value_type>
void quick_sort(IterT first, IterT last) {
if (std::distance(first, last) > 1) {
IterT prev_last = std::prev(last);
IterT cut = std::partition(first, prev_last, [prev_last](T v) { return v < *prev_last; });
std::iter_swap(cut, prev_last);
quick_sort(first, cut);
quick_sort(cut, last);
}
}
```
> 一点优化（Liam Huang）：通过将 `if` 改为 `while` 同时修改 `last` 迭代器的值，可以节省一半递归调用的开销。
```cpp
template <typename IterT, typename T = typename std::iterator_traits<IterT>::value_type>
void quick_sort(IterT first, IterT last) {
while (std::distance(first, last) > 1) {
IterT prev_last = std::prev(last);
IterT cut = std::partition(first, prev_last, [prev_last](T v) { return v < *prev_last; });
std::iter_swap(cut, prev_last);
quick_sort(cut, last);
last = cut;
}
}
```
如果不要求空间复杂度，分区函数实现起来很容易。
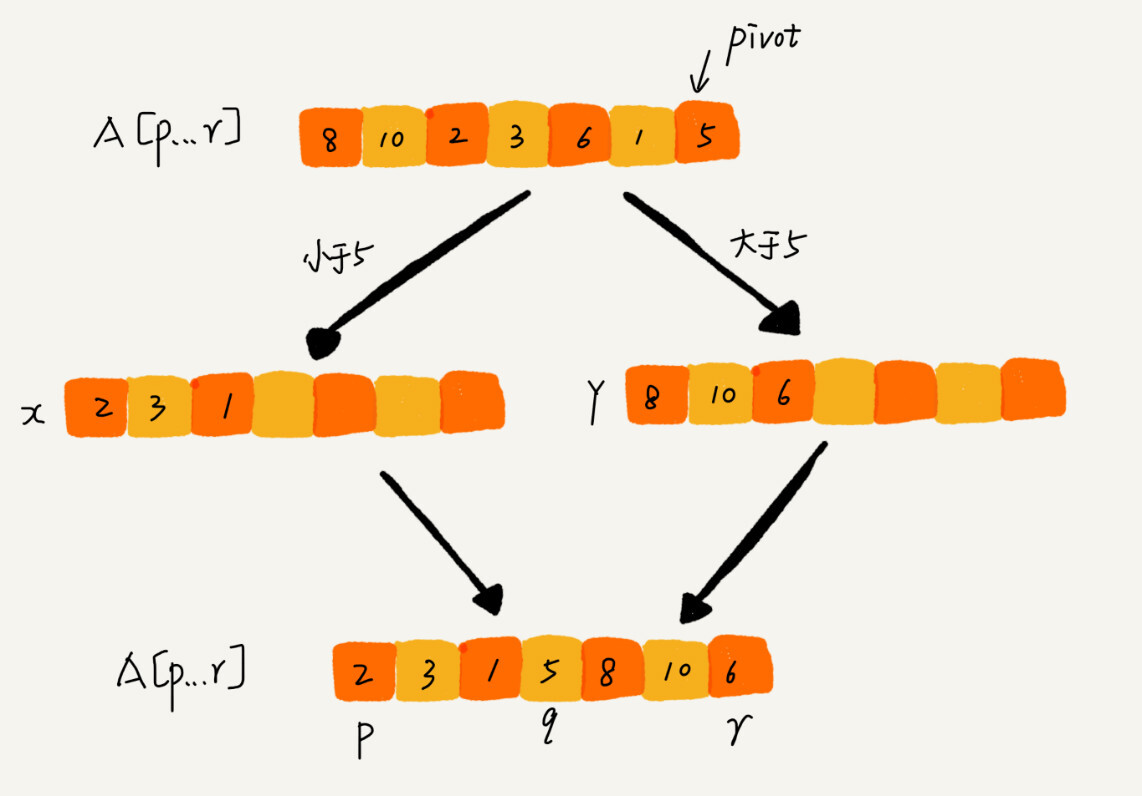
![非原地分区](https://static001.geekbang.org/resource/image/66/dc/6643bc3cef766f5b3e4526c332c60adc.jpg)
若要求原地分区，则不那么容易了。下面的实现实现了原地分区函数，并且能将所有相等的主元排在一起。
```cpp
template <typename BidirIt,
typename T = typename std::iterator_traits<BidirIt>::value_type,
typename Compare = std::less<T>>
std::pair<BidirIt, BidirIt> inplace_partition(BidirIt first,
BidirIt last,
const T& pivot,
Compare comp = Compare()) {
BidirIt last_less, last_greater, first_equal, last_equal;
for (last_less = first, last_greater = first, first_equal = last;
last_greater != first_equal; ) {
if (comp(*last_greater, pivot)) {
std::iter_swap(last_greater++, last_less++);
} else if (comp(pivot, *last_greater)) {
++last_greater;
} else { // pivot == *last_greater
std::iter_swap(last_greater, --first_equal);
}
}
const auto cnt = std::distance(first_equal, last);
std::swap_ranges(first_equal, last, last_less);
first_equal = last_less;
last_equal = first_equal + cnt;
return {first_equal, last_equal};
}
```
### 算法分析
* 稳定性
* 由于 `inplace_partition` 使用了大量 `std::iter_swap` 操作，所以不是稳定排序
* 时间复杂度
* 定义 $T(n)$ 表示问题规模为 $n$ 时算法的耗时，
* 有递推公式：$T(n) = 2T(n/2) + n$（假定每次分割都是均衡分割）
* 展开得 $T(n) = 2^{k}T(1) + k * n$
* 考虑 $k$ 是递归深度，它的值是 $\log_2 n$，因此 $T(n) = n + n\log_2 n$
* 因此，快速排序的时间复杂度为 $\Theta(n\log n)$
* 空间复杂度
* 一般来说，空间复杂度是 $\Theta(1)$，因此是原地排序算法
## 开篇问题
* 分区，看前半段元素数量
* 前半段元素数量 < K，对后半段进行分区
* 前半段元素数量 > K，对前半段进行分区
* 前半段元素数量 = K，前半段末位元素即是所求

0
notes/13_sorts/.gitkeep Normal file
View File

77
notes/13_sorts/readme.md Normal file
View File

@ -0,0 +1,77 @@
# 线性排序
## 开篇问题
如何按年龄给 100 万用户排序？
## 桶排序（Bucket Sort）
算法思想：
* 按待排序数据的 key 分有序桶
* 桶内排序
* 有序桶依次输出
![桶排序示例](https://static001.geekbang.org/resource/image/98/ae/987564607b864255f81686829503abae.jpg)
### 算法分析
* 时间复杂度 $O(n)$
* $n$ 个元素，分 $m$ 个有序桶，每个桶里平均 $k = n / m$ 个元素
* 桶内快排，复杂度 $O(k \log k)$，$m$ 个桶一共 $O(n \log k)$
* 当 $m$ 接近 $n$，例如当 $k = 4$ 时，这个复杂度近似 $O(n)$
* 使用条件
* 数据易于分如有序桶
* 数据在各个有序桶之间分布均匀
* 适合外部排序——数据不全部载入磁盘
## 计数排序（Counting Sort）
计数排序可以视作是桶排序的一个特殊情况：
* 数据的取值范围很小
* 每个分桶内的元素 key 值都一样
此时，由于分桶内的元素 key 值都一样，所以桶内的排序操作可以省略，以及桶的编号本身就能记录桶内元素的值。因此，算法只需遍历一遍所有的数据，统计每个取值上有多少元素即可。这个过程时间复杂度是 $O(n)$。
* 假设待排序的数组 `A = {2, 5, 3, 0, 2, 3, 0, 3}`，我们有计数数组 `C = {2, 0, 2, 3, 0, 1}`
接下来，我们要对 `C` 进行计数操作，具体来说，对从下标为 1 的元素开始累加 `C[i] += C[i - 1]`。
* 计数累加 `C = {2, 2, 4, 7, 7, 8}`
此时，`C` 中的元素表示「小于等于下标的元素的个数」。接下来，我们从尾至头扫描待排序数组 `A`，将其中元素依次拷贝到输出数组 `R` 的相应位置。我们注意到，`A[7] = 3` 而 `C[3] == 4` 。这意味着，待排序的数组中，包括 3 本身在内，不超过 3 的元素共有 4 个。因此，我们可以将这个 3 放置在 `R[C[3] - 1]` 的位置，而后将 `C[3]` 的计数减一——这是由于待排序数组中未处理的部分，不超过 3 的元素现在只剩下 3 个了。如此遍历整个待排序数组 `A`，即可得到排序后的结果 `R`。
![计数排序示例](https://static001.geekbang.org/resource/image/1d/84/1d730cb17249f8e92ef5cab53ae65784.jpg)
### 算法分析
* 时间复杂度
* $n$ 个元素，最大值是 $k$，分 $k$ 个「桶」；时间复杂度 $O(n)$
* 桶内计数累加；时间复杂度 $O(k)$
* 摆放元素；时间复杂度 $O(n)$
* 当 $k < n$ 时，总体时间复杂度是 $O(n)$
* 使用条件
* $k < n$
* 待排序数据的 key 是非负整数
## 基数排序（Radix Sort）
基数排序适用于等长数据的排序。对于不等长数据，可以在较短的数据后面做 padding，使得数据等长。
* 先就 least significant digit 进行稳定排序——通常可以用桶排序或者计数排序；时间复杂度 $O(n)$
* 而后依次向 greatest significant digit 移动，进行稳定排序
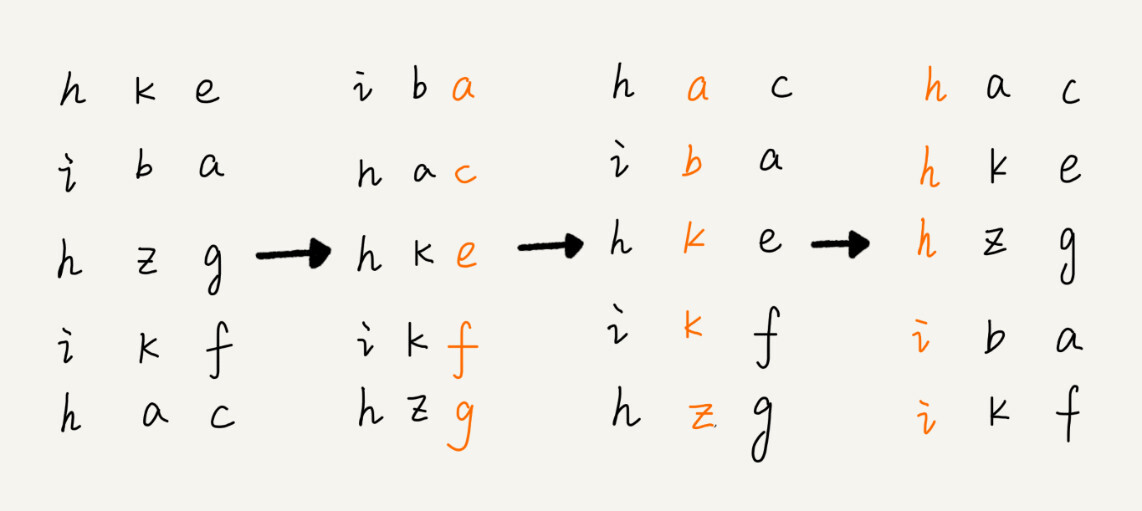
![基数排序示例](https://static001.geekbang.org/resource/image/df/0c/df0cdbb73bd19a2d69a52c54d8b9fc0c.jpg)
### 算法分析
* 时间复杂度
* 对每一位的排序时间复杂度是 $O(n)$
* 总共 $k$ 位，因此总的时间复杂度是 $O(kn)$；考虑到 $k$ 是常数，因此总的时间复杂度是 $O(n)$
* 使用条件
* 等长数据
## 解答开篇
桶排序。

0
notes/14_sorts/.gitkeep Normal file
View File

10
notes/14_sorts/readme.md Normal file
View File

@ -0,0 +1,10 @@
# 排序优化
## 如何取舍排序算法？
* 排序规模小 —— $O(n^2)$ 的算法（通常是插排）
* 排序规模大 —— $O(n\log n)$ 的算法（通常不用归并排序）
## 如何优化快速排序？
参考：[谈谈内省式排序算法](https://liam.page/2018/08/29/introspective-sort/)

0
notes/15_bsearch/.gitkeep Normal file
View File

23
notes/15_bsearch/readme.md Normal file
View File

@ -0,0 +1,23 @@
# 二分查找（上）
## 算法描述
二分查找（Binary Search）也叫折半查找，是针对有序数据集合的查找算法。其描述十分简单：
* 折半取中，判断元素与目标元素的大小关系
* 小于——往前继续折半
* 大于——往后继续折半
* 等于——返回
关于它的复杂度分析，参见[谈谈基于比较的排序算法的复杂度下界](https://liam.page/2018/08/28/lower-bound-of-comparation-based-sort-algorithm/)中的相关信息。它的复杂度是 $O(\log n)$。
## $O(\log n)$ 的惊人之处
在 42 亿个数据中用二分查找一个数据，最多需要比较 32 次。
## 适用场景
* 依赖顺序表结构
* 数据本身必须有序
* 数据量相对比较元素的开销要足够大——不然遍历即可
* 数据量相对内存空间不能太大——不然顺序表装不下

0
notes/16_bsearch/.gitkeep Normal file
View File

100
notes/16_bsearch/readme.md Normal file
View File

@ -0,0 +1,100 @@
# 二分查找（下）
本节课讨论二分的各种变体。实际上在针对上一节的代码中，已经实现了两个变体。本次实现四个变体：
* 第一个等于给定值的元素
* 最后一个等于给定值的元素
* 第一个不小于给定值的元素
* 最后一个不大于给定值的元素
```cpp
/**
* Created by Liam Huang (Liam0205) on 2018/10/26.
*/
#ifndef BSEARCH_BSEARCH_VARIENTS_HPP_
#define BSEARCH_BSEARCH_VARIENTS_HPP_
#include <iterator>
#include <functional>
enum class BsearchPolicy { UNSPECIFIED, FIRST, LAST, FIRST_NOT_LESS, LAST_NOT_GREATER };
// Liam Huang: The algorithm works right with iterators that meet the ForwardIterator requirement,
// but with a bad time complexity. For better performance, iterators should meet
// the RandomAccessIterator requirement.
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare>
IterT bsearch(IterT first,
IterT last,
ValueT target,
Compare comp,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
IterT result = last;
while (std::distance(first, last) > 0) {
IterT mid = first + std::distance(first, last) / 2;
if (policy == BsearchPolicy::FIRST_NOT_LESS) {
if (!comp(*mid, target)) {
if (mid == first or comp(*(mid - 1), target)) {
result = mid;
break;
} else {
last = mid;
}
} else {
first = mid + 1;
}
} else if (policy == BsearchPolicy::LAST_NOT_GREATER) {
if (comp(target, *mid)) {
last = mid;
} else {
if (std::distance(mid, last) == 1 or comp(target, *(mid + 1))) {
result = mid;
break;
} else {
first = mid + 1;
}
}
} else { // policy == UNSPECIFIED or FIRST or LAST
if (comp(*mid, target)) {
first = mid + 1;
} else if (comp(target, *mid)) {
last = mid;
} else { // equal
if (policy == BsearchPolicy::FIRST) {
if (mid == first or comp(*(mid - 1), *mid)) {
result = mid;
break;
} else {
last = mid;
}
} else if (policy == BsearchPolicy::LAST) {
if (std::distance(mid, last) == 1 or comp(*mid, *(mid + 1))) {
result = mid;
break;
} else {
first = mid + 1;
}
} else {
result = mid;
break;
}
}
}
}
return result;
}
template <typename IterT,
typename ValueT = typename std::iterator_traits<IterT>::value_type,
typename Compare = std::less<ValueT>>
IterT bsearch(IterT first,
IterT last,
ValueT target,
BsearchPolicy policy = BsearchPolicy::UNSPECIFIED) {
return bsearch(first, last, target, Compare(), policy);
}
#endif // BSEARCH_BSEARCH_VARIENTS_HPP_
```

0
notes/17_skiplist/.gitkeep Normal file
View File

43
notes/17_skiplist/readme.md Normal file
View File

@ -0,0 +1,43 @@
# 跳表（Skip List）
支持快速地：
* 插入
* 删除
* 查找
某些情况下，跳表甚至可以替代红黑树（Red-Black tree）。Redis 当中的有序集合（Sorted Set）是用跳表实现的。
## 跳表的结构
跳表是对链表的改进。对于单链表来说，即使内容是有序的，查找具体某个元素的时间复杂度也要达到 $O(n)$。对于二分查找来说，由于链表不支持随机访问，根据 `first` 和 `last` 确定 `cut` 时，必须沿着链表依次迭代 `std::distance(first, last) / 2` 步；特别地，计算 `std::(first, last)` 本身，就必须沿着链表迭代才行。此时，二分查找的效率甚至退化到了 $O(n \log n)$，甚至还不如顺序遍历。
![单链表查找示例](https://static001.geekbang.org/resource/image/e1/6d/e18303fcedc068e5a168de04df956f6d.jpg)
跳表的核心思想是用空间换时间，构建足够多级数的索引，来缩短查找具体值的时间开销。
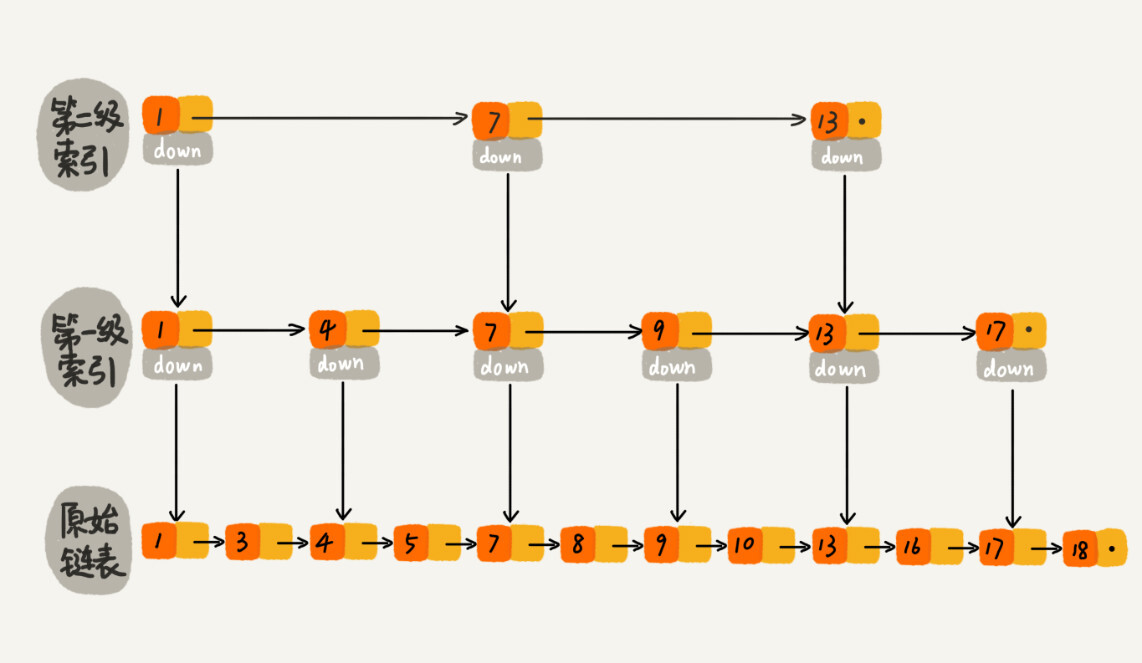
![具有二级索引的跳表示例](https://static001.geekbang.org/resource/image/49/65/492206afe5e2fef9f683c7cff83afa65.jpg)
例如对于一个具有 64 个有序元素的五级跳表，查找起来的过程大约如下图所示。
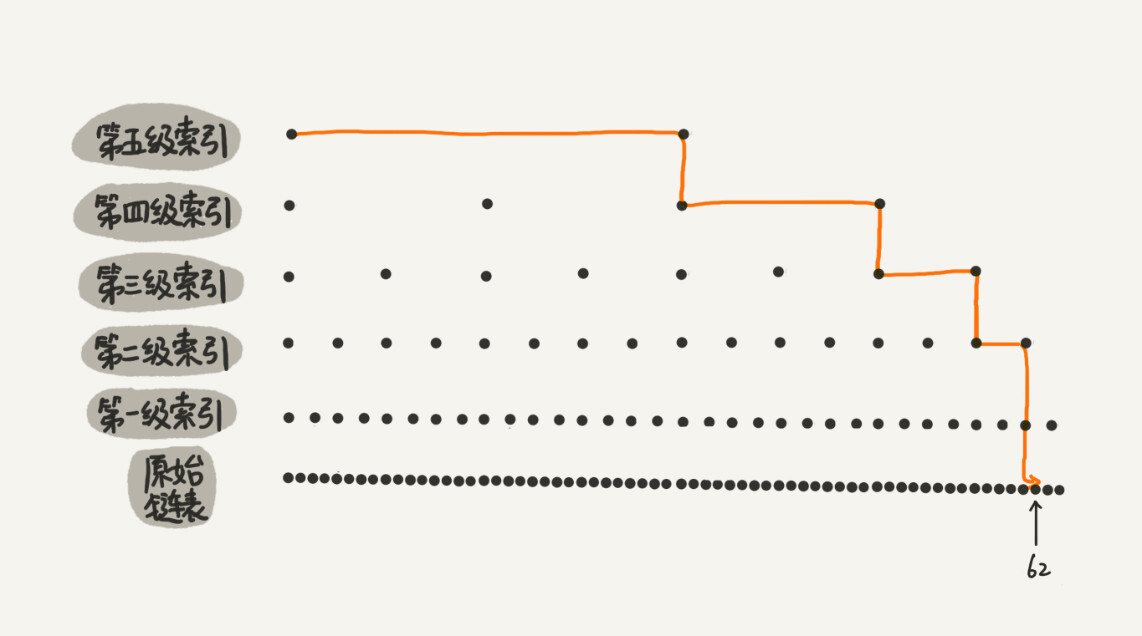
![五级跳表示例](https://static001.geekbang.org/resource/image/46/a9/46d283cd82c987153b3fe0c76dfba8a9.jpg)
## 复杂度分析
对于一个每一级索引的跨度是下一级索引 $k$ 倍的跳表，每一次 `down` 操作，相当于将搜索范围缩小到「剩余的可能性的 $1 / k$」。因此，查找具体某个元素的时间复杂度大约需要 $\lfloor \log_k n\rfloor + 1$ 次操作；也就是说时间复杂度是 $O(\log n)$。
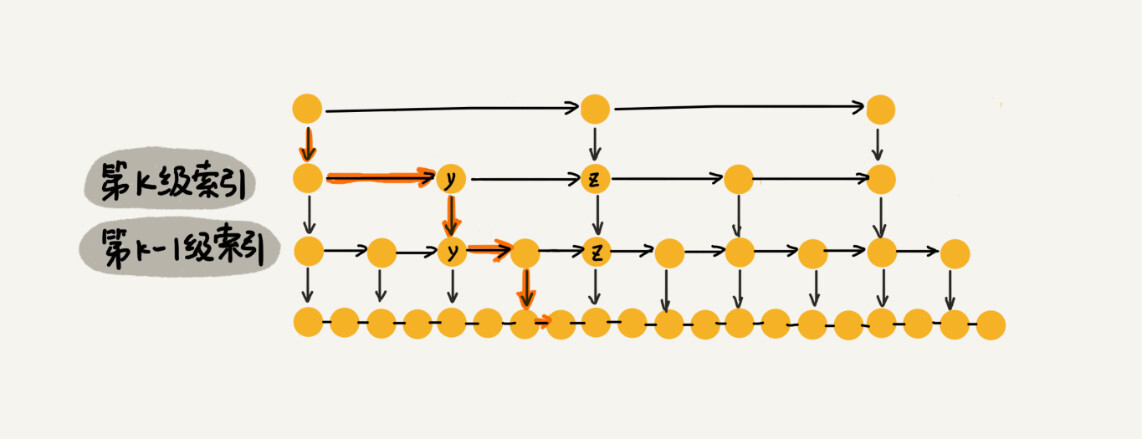
![跳表查询过程示例](https://static001.geekbang.org/resource/image/d0/0c/d03bef9a64a0368e6a0d23ace8bd450c.jpg)
前面说了，跳表是一种用空间换时间的数据结构。因此它的空间复杂度一定不小。我们考虑原链表有 $n$ 个元素，那么第一级索引就有 $n / k$ 个元素，剩余的索引依次有 $n / k^2$, $n / k^3$, ..., $1$ 个元素。总共的元素个数是一个等比数列求和问题，它的值是 $\frac{n - 1}{k - 1}$。可见，不论 $k$ 是多少，跳表的空间复杂度都是 $O(n)$；但随着 $k$ 的增加，实际需要的额外节点数会下降。
## 高效地插入和删除
对于链表来说，插入或删除一个给定结点的时间复杂度是 $O(1)$。因此，对于跳表来说，插入或删除某个结点，其时间复杂度完全依赖于查找这类结点的耗时。而我们知道，在跳表中查找某个元素的时间复杂度是 $O(\log n)$。因此，在跳表中插入或删除某个结点的时间复杂度是 $O(\log n)$。
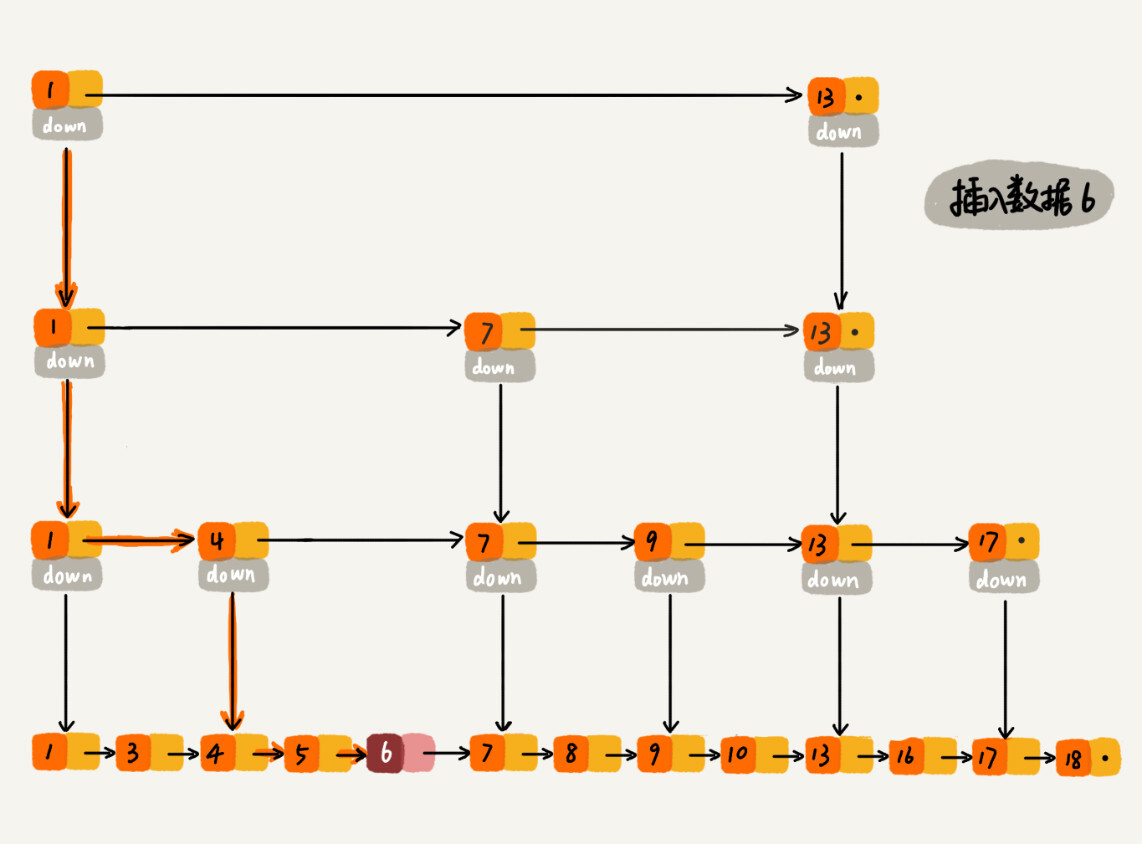
![在跳表中插入一个元素](https://static001.geekbang.org/resource/image/65/6c/65379f0651bc3a7cfd13ab8694c4d26c.jpg)
## 跳表索引的动态更新
为了维护跳表的结构，在不断插入数据的过程中，有必要动态维护跳表的索引结构。一般来说，可以采用随机层级法。具体来说是引入一个输出整数的随机函数。当随机函数输出 $K$，则更新从第 $1$ 级至第 $K$ 级的索引。为了保证索引结构和数据规模大小的匹配，一般采用二项分布的随机函数。
![在跳表中插入一个元素并更新索引](https://static001.geekbang.org/resource/image/a8/a7/a861445d0b53fc842f38919365b004a7.jpg)

47
php/12_sort/quicksort.php Normal file
View File

@ -0,0 +1,47 @@
<?php
function quickSort(array &$a)
{
$n = count($a);
quickSortInternally($a, 0, $n-1);
}
function quickSortInternally(array &$a, int $l, int $r)
{
if ($l >= $r) return;
$q = partition($a, $l, $r);
quickSortInternally($a, $l, $q-1);
quickSortInternally($a, $q+1, $r);
}
function partition(&$a, $l, $r): int
{
$pivot = $a[$r];
$i = $l;
for ($j = $l; $j < $r; ++$j) {
if ($a[$j] < $pivot) {
[$a[$j], $a[$i]] = [$a[$i], $a[$j]];
++$i;
}
}
[$a[$r], $a[$i]] = [$a[$i], $a[$r]];
return $i;
}
$a1 = [1,4,6,2,3,5,4];
$a2 = [2, 2, 2, 2];
$a3 = [4, 3, 2, 1];
$a4 = [5, -1, 9, 3, 7, 8, 3, -2, 9];
quickSort($a1);
print_r($a1);
quickSort($a2);
print_r($a2);
quickSort($a3);
print_r($a3);
quickSort($a4);
print_r($a4);

BIN
python/.vs/slnx.sqlite Normal file
View File

Binary file not shown.

75
python/08_stack/simple_browser.py Normal file
View File

@ -0,0 +1,75 @@
"""
a simple browser realize
Author: zhenchao.zhu
解答：我们使用两个栈，X 和 Y，我们把首次浏览的页面依次压入栈 X，当点击后退按钮时，再依次从栈 X 中出栈，
并将出栈的数据依次放入栈 Y。当我们点击前进按钮时，我们依次从栈 Y 中取出数据，放入栈 X 中。
当栈 X 中没有数据时，那就说明没有页面可以继续后退浏览了。当栈 Y 中没有数据，
那就说明没有页面可以点击前进按钮浏览了。
"""
import sys
# 引用当前文件夹下的single_linked_list
sys.path.append('linked_stack.py')
from linked_stack import LinkedStack
#from .linked_stack import LinkedStack
class NewLinkedStack(LinkedStack):
def is_empty(self):
return not self._top
class Browser():
def __init__(self):
self.forward_stack = NewLinkedStack()
self.back_stack = NewLinkedStack()
def can_forward(self):
if self.back_stack.is_empty():
return False
return True
def can_back(self):
if self.forward_stack.is_empty():
return False
return True
def open(self, url):
print("Open new url %s" % url, end="\n")
self.forward_stack.push(url)
def back(self):
if self.forward_stack.is_empty():
return
top = self.forward_stack.pop()
self.back_stack.push(top)
print("back to %s" % top, end="\n")
def forward(self):
if self.back_stack.is_empty():
return
top = self.back_stack.pop()
self.forward_stack.push(top)
print("forward to %s" % top, end="\n")
if __name__ == '__main__':
browser = Browser()
browser.open('a')
browser.open('b')
browser.open('c')
if browser.can_back():
browser.back()
if browser.can_forward():
browser.forward()
browser.back()
browser.back()
browser.back()

91
python/17_skiplist/skip_list.py Normal file
View File

@ -0,0 +1,91 @@
"""
An implementation of skip list.
The list stores positive integers without duplicates.
跳表的一种实现方法。
跳表中储存的是正整数，并且储存的是不重复的。
Author: Wenru
"""
from typing import Optional
import random
class ListNode:
def __init__(self, data: Optional[int] = None):
self._data = data
self._forwards = [] # Forward pointers
class SkipList:
_MAX_LEVEL = 16
def __init__(self):
self._level_count = 1
self._head = ListNode()
self._head._forwards = [None] * type(self)._MAX_LEVEL
def find(self, value: int) -> Optional[ListNode]:
p = self._head
for i in range(self._level_count - 1, -1, -1): # Move down a level
while p._forwards[i] and p._forwards[i]._data < value:
p = p._forwards[i] # Move along level
return p._forwards[0] if p._forwards[0] and p._forwards[0]._data == value else None
def insert(self, value: int):
level = self._random_level()
if self._level_count < level: self._level_count = level
new_node = ListNode(value)
new_node._forwards = [None] * level
update = [self._head] * level # update is like a list of prevs
p = self._head
for i in range(level - 1, -1, -1):
while p._forwards[i] and p._forwards[i]._data < value:
p = p._forwards[i]
update[i] = p # Found a prev
for i in range(level):
new_node._forwards[i] = update[i]._forwards[i] # new_node.next = prev.next
update[i]._forwards[i] = new_node # prev.next = new_node
def delete(self, value):
update = [None] * self._level_count
p = self._head
for i in range(self._level_count - 1, -1, -1):
while p._forwards[i] and p._forwards[i]._data < value:
p = p._forwards[i]
update[i] = p
if p._forwards[0] and p._forwards[0]._data == value:
for i in range(self._level_count - 1, -1, -1):
if update[i]._forwards[i] and update[i]._forwards[i]._data == value:
update[i]._forwards[i] = update[i]._forwards[i]._forwards[i] # Similar to prev.next = prev.next.next
def _random_level(self, p: float = 0.5) -> int:
level = 1
while random.random() < p and level < type(self)._MAX_LEVEL:
level += 1
return level
def __repr__(self) -> str:
values = []
p = self._head
while p._forwards[0]:
values.append(str(p._forwards[0]._data))
p = p._forwards[0]
return "->".join(values)
if __name__ == "__main__":
l = SkipList()
for i in range(10):
l.insert(i)
print(l)
p = l.find(7)
print(p._data)
l.delete(3)
print(l)

18
scala/15_bsearch/BSearch.scala Normal file
View File

@ -0,0 +1,18 @@
object BSearch {
def search(nums: Array[Int], target: Int): Int = {
var low = 0
var high = nums.length - 1
while(low <= high){
val mid = low + ((high - low) >> 2)
if(nums(mid) > target){
high = mid - 1
} else if (nums(mid) < target){
low = mid + 1
} else {
return mid
}
}
return -1
}
}

20
scala/15_bsearch/BSearchRecursive.scala Normal file
View File

@ -0,0 +1,20 @@
object BSearchRecursive {
def search(nums: Array[Int], target: Int): Int = {
return searchInternal(nums, target, 0, nums.length - 1)
}
def searchInternal(nums:Array[Int], target: Int, low: Int, high: Int): Int = {
if(low <= high){
val mid = low + ((high - low) >> 2)
if(nums(mid) > target){
searchInternal(nums, target, low, mid - 1)
} else if (nums(mid) < target){
searchInternal(nums, target, mid + 1, high)
} else {
return mid
}
}else{
return -1
}
}
}

128
swift/06_linkedlist/SinglyLinkedList.swift Normal file
View File

@ -0,0 +1,128 @@
//
// Created by Jiandan on 2018/10/12.
// Copyright © 2018 Jiandan. All rights reserved.
//
import Foundation
class Node<T> {
var value: T?
var next: Node?
init(){}
init(value: T) {
self.value = value
}
}
/// 单链表
/// 实现插入、删除、查找操作
class List<Element: Equatable> {
private var dummy = Node<Element>() // 哨兵结点，不存储数据
var size: Int {
var num = 0
var tmpNode = dummy.next
while tmpNode != nil {
num += 1
tmpNode = tmpNode!.next
}
return num
}
var isEmpty: Bool { return size > 0 }
/// find node with value
func node(with value: Element) -> Node<Element>? {
var node = dummy.next
while node != nil {
if node!.value == value {
return node
}
node = node!.next
}
return nil
}
// 约定：链表的 index 从 1 开始
func node(at index: Int) -> Node<Element>? {
var num = 1
var node = dummy.next
while node != nil {
if num == index {
return node
}
node = node!.next
num += 1
}
return nil
}
func insertToHead(value: Element) {
let newNode = Node(value: value)
return insertToHead(node: newNode)
}
func insertToHead(node: Node<Element>) {
node.next = dummy.next
dummy.next = node
}
func insert(after node: Node<Element>, newValue: Element) {
let newNode = Node(value: newValue)
return insert(after: node, newNode: newNode)
}
func insert(after node: Node<Element>, newNode: Node<Element>) {
newNode.next = node.next
node.next = newNode
}
func insert(before node: Node<Element>, newValue: Element) {
let newNode = Node(value: newValue)
return insert(before: node, newNode: newNode)
}
func insert(before node: Node<Element>, newNode: Node<Element>) {
var lastNode = dummy
var tmpNode = dummy.next
while tmpNode != nil {
if tmpNode === node {
newNode.next = tmpNode
lastNode.next = newNode
break
}
lastNode = tmpNode!
tmpNode = tmpNode!.next
}
}
func delete(node: Node<Element>) {
var lastNode = dummy
var tmpNode = dummy.next
while tmpNode != nil {
if tmpNode === node {
lastNode.next = tmpNode!.next
break
}
lastNode = tmpNode!
tmpNode = tmpNode!.next
}
}
/// 删除首个 value 符合要求的结点
func delete(value: Element) {
var lastNode = dummy
var tmpNode = dummy.next
while tmpNode != nil {
if tmpNode!.value == value {
lastNode.next = tmpNode!.next
break
}
lastNode = tmpNode!
tmpNode = tmpNode!.next
}
}
}

111
swift/07_linkedlist/LinkedListAlgo.swift Normal file
View File

@ -0,0 +1,111 @@
//
// Created by Jiandan on 2018/10/13.
// Copyright (c) 2018 Jiandan. All rights reserved.
//
/**
* 1) 单链表反转
* 2) 链表中环的检测
* 3) 两个有序的链表合并
* 4) 删除链表倒数第n个结点
* 5) 求链表的中间结点
*/
import Foundation
/// 单链表反转
func reverseSinglyLinkedList<Element>(head: Node<Element>) -> Node<Element>? {
var reverseHead: Node<Element>?, currentNode: Node<Element>?, prevNode: Node<Element>?
currentNode = head
while currentNode != nil {
let nextNode = currentNode!.next
if nextNode == nil {
reverseHead = currentNode
}
currentNode!.next = prevNode
prevNode = currentNode
currentNode = nextNode
}
return reverseHead
}
/// 检测环
func hasCircle<Element>(head: Node<Element>) -> Bool {
var fast = head.next
var slow: Node<Element>? = head
while fast != nil {
if fast === slow {
return true
}
fast = fast!.next?.next
slow = slow!.next
}
return false
}
/// 两个有序的链表合并
func mergeSortedLists<Element: Comparable>(headA: Node<Element>?, headB: Node<Element>?) -> Node<Element>? {
guard let headA = headA else {
return headB
}
guard let headB = headB else {
return headA
}
var head: Node<Element>?, tail: Node<Element>?
var nodeA: Node<Element>? = headA, nodeB: Node<Element>? = headB
if nodeA!.value! < nodeB!.value! {
head = nodeA
nodeA = nodeA!.next
} else {
head = nodeB
nodeB = nodeB!.next
}
tail = head
while nodeA != nil, nodeB != nil {
if nodeA!.value! < nodeB!.value! {
tail!.next = nodeA
nodeA = nodeA!.next
} else {
tail!.next = nodeB
nodeB = nodeB!.next
}
tail = tail!.next
}
if nodeA != nil {
tail?.next = nodeA
} else {
tail?.next = nodeB
}
return head
}
/// 删除倒数第n个结点
func deleteNode<Element>(at lastNum: Int, in head: Node<Element>) {
var slow: Node<Element>? = head
var fast: Node<Element>? = head
var num = 1
while fast != nil, num < lastNum {
fast = fast!.next
num += 1
}
var prevNode: Node<Element>?
while fast != nil {
prevNode = slow
fast = fast!.next
slow = slow!.next
}
prevNode?.next = slow?.next
}
/// 求链表的中间结点
func halfNode<Element>(in head: Node<Element>) -> Node<Element>? {
var slow: Node<Element>? = head
var fast: Node<Element>? = head
while fast?.next != nil, fast?.next?.next != nil {
fast = fast!.next?.next
slow = slow!.next
}
return slow
}

27
swift/08_stack/Browser.swift Normal file
View File

@ -0,0 +1,27 @@
//
// Created by Jiandan on 2018/10/12.
// Copyright (c) 2018 Jiandan. All rights reserved.
//
import Foundation
struct Page {
/// 存储前进 url
private var forwardArray = [String]()
/// 存储后退 url
private var backArray = [String]()
var currentURL: String? { return forwardArray.last }
init(url: String) {
forwardArray.append(url)
}
/// 前进
mutating func goForward(url: String) {
forwardArray.append(url)
}
/// 后退
mutating func goBack() {
backArray.append(forwardArray.popLast()!)
}
}

27
swift/08_stack/BrowserDemo.swift Normal file
View File

@ -0,0 +1,27 @@
//
// Created by Jiandan on 2018/10/12.
// Copyright (c) 2018 Jiandan. All rights reserved.
//
import Foundation
struct Page {
/// 存储前进 url
private var forwardArray = [String]()
/// 存储后退 url
private var backArray = [String]()
var currentURL: String { return forwardArray.last! }
init(url: String) {
forwardArray.append(url)
}
/// 前进
mutating func goForward(url: String) {
forwardArray.append(url)
}
/// 后退
mutating func goBack() {
backArray.append(forwardArray.popLast()!)
}
}

21
swift/08_stack/Stack.swift Normal file
View File

@ -0,0 +1,21 @@
//
// Created by Jiandan on 2018/10/12.
// Copyright (c) 2018 Jiandan. All rights reserved.
//
import Foundation
protocol Stack {
/// 持有的数据类型
associatedtype Element
/// 是否为空
var isEmpty: Bool { get }
/// 队列大小
var size: Int { get }
/// 返回队列头部元素
var peek: Element? { get }
/// 入栈
mutating func push(newElement: Element) -> Bool
/// 出栈
mutating func pop() -> Element?
}

37
swift/08_stack/StackBasedOnLinkedList.swift Normal file
View File

@ -0,0 +1,37 @@
//
// Created by Jiandan on 2018/10/12.
// Copyright (c) 2018 Jiandan. All rights reserved.
//
import Foundation
struct StackBasedOnLinkedList<Element>: Stack {
private var head = Node<Element>() // 哨兵结点，不存储内容
// MARK: Protocol: Stack
var isEmpty: Bool { return head.next == nil }
var size: Int {
var count = 0
while head.next != nil {
count += 1
}
return count
}
var peek: Element? { return head.next?.value }
func push(newElement: Element) -> Bool {
let node = Node(value: newElement)
node.next = head.next
head.next = node
return true
}
func pop() -> Element? {
let node = head.next
head.next = node?.next
return node?.value
}
}

8
swift/09_queue/ArrayQueue.swift
View File

@ -6,9 +6,7 @@
import Foundation
/// 用数组实现的队列
struct ArrayQueue<T>: Queue {
typealias Element = T
struct ArrayQueue<Element>: Queue {
/// 数组
private var items: [Element]
/// 数组最大长度
@ -78,9 +76,7 @@ struct ArrayQueue<T>: Queue {
/// 使用2个数组实现无界队列，用到 Swift 中 Array 较多的方法
/// 来源：《iOS 面试之道》（故胤道长，唐巧）
struct ArrayQueue2<T>: Queue {
typealias Element = T
struct ArrayQueue2<Element>: Queue {
/// 输入数组，主要负责入队
var inArray = [Element]()
/// 输出数组，主要负责出队

4
swift/09_queue/CircularQueue.swift
View File

@ -6,9 +6,7 @@
import Foundation
/// 循环队列
struct CircularQueue<T>: Queue {
typealias Element = T
struct CircularQueue<Element>: Queue {
/// 数组
private var items: [Element]
/// 数组最大长度

20
swift/09_queue/QueueBasedOnLinkedList.swift
View File

@ -5,18 +5,7 @@
import Foundation
class Node<T> {
var value: T?
var next: Node?
init(value: T) {
self.value = value
}
}
struct QueueBasedOnLinkedList<T>: Queue {
typealias Element = T
struct QueueBasedOnLinkedList<Element>: Queue {
/// 队首
var head: Node<Element>?
/// 队尾
@ -27,10 +16,15 @@ struct QueueBasedOnLinkedList<T>: Queue {
var isEmpty: Bool { return head == nil }
var size: Int {
var count = 0
if isEmpty {
return 0
}
var count = 1 // head 本身算一个
while head?.next != nil {
count += 1
}
return count
}